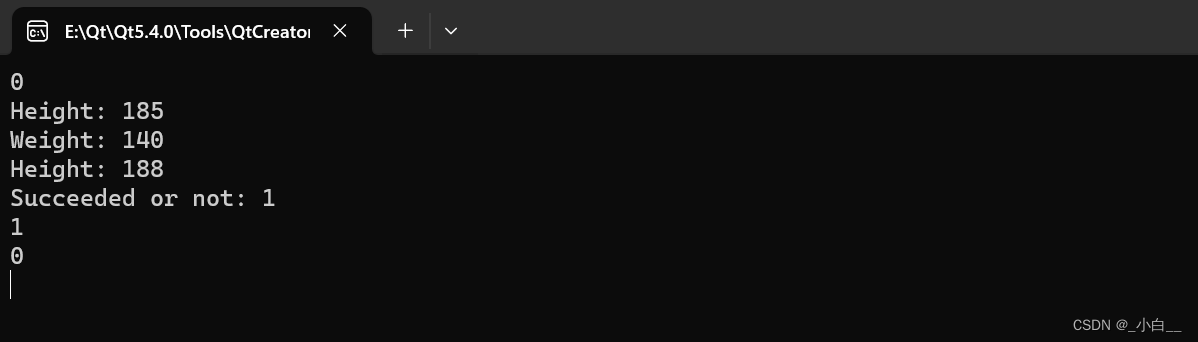
1 文本格式
// C++ program to implement Quick Hull algorithm
// to find convex hull.
#include<bits/stdc++.h>
using namespace std;
// iPair is integer pairs
#define iPair pair<int, int>
// Stores the result (points of convex hull)
set<iPair> hull;
// Returns the side of point p with respect to line
// joining points p1 and p2.
int findSide(iPair p1, iPair p2, iPair p)
{
int val = (p.second - p1.second) * (p2.first - p1.first) -
(p2.second - p1.second) * (p.first - p1.first);
if (val > 0)
return 1;
if (val < 0)
return -1;
return 0;
}
// returns a value proportional to the distance
// between the point p and the line joining the
// points p1 and p2
int lineDist(iPair p1, iPair p2, iPair p)
{
return abs((p.second - p1.second) * (p2.first - p1.first) -
(p2.second - p1.second) * (p.first - p1.first));
}
// End points of line L are p1 and p2. side can have value
// 1 or -1 specifying each of the parts made by the line L
void quickHull(iPair a[], int n, iPair p1, iPair p2, int side)
{
int ind = -1;
int max_dist = 0;
// finding the point with maximum distance
// from L and also on the specified side of L.
for (int i = 0; i < n; i++)
{
int temp = lineDist(p1, p2, a[i]);
if (findSide(p1, p2, a[i]) == side && temp > max_dist)
{
ind = i;
max_dist = temp;
}
}
// If no point is found, add the end points
// of L to the convex hull.
if (ind == -1)
{
hull.insert(p1);
hull.insert(p2);
return;
}
// Recur for the two parts divided by a[ind]
quickHull(a, n, a[ind], p1, -findSide(a[ind], p1, p2));
quickHull(a, n, a[ind], p2, -findSide(a[ind], p2, p1));
}
void printHull(iPair a[], int n)
{
// a[i].second -> y-coordinate of the ith point
if (n < 3)
{
cout << "Convex hull not possible\n";
return;
}
// Finding the point with minimum and
// maximum x-coordinate
int min_x = 0, max_x = 0;
for (int i = 1; i < n; i++)
{
if (a[i].first < a[min_x].first)
min_x = i;
if (a[i].first > a[max_x].first)
max_x = i;
}
// Recursively find convex hull points on
// one side of line joining a[min_x] and
// a[max_x]
quickHull(a, n, a[min_x], a[max_x], 1);
// Recursively find convex hull points on
// other side of line joining a[min_x] and
// a[max_x]
quickHull(a, n, a[min_x], a[max_x], -1);
cout << "The points in Convex Hull are:\n";
while (!hull.empty())
{
cout << "(" << (*hull.begin()).first << ", "
<< (*hull.begin()).second << ") ";
hull.erase(hull.begin());
}
}
// Driver code
int main()
{
iPair a[] = { {0, 3}, {1, 1}, {2, 2}, {4, 4},
{0, 0}, {1, 2}, {3, 1}, {3, 3} };
int n = sizeof(a) / sizeof(a[0]);
printHull(a, n);
return 0;
}
2 代码格式
// C++ program to implement Quick Hull algorithm
// to find convex hull.
#include<bits/stdc++.h>
using namespace std;
// iPair is integer pairs
#define iPair pair<int, int>
// Stores the result (points of convex hull)
set<iPair> hull;
// Returns the side of point p with respect to line
// joining points p1 and p2.
int findSide(iPair p1, iPair p2, iPair p)
{
int val = (p.second - p1.second) * (p2.first - p1.first) -
(p2.second - p1.second) * (p.first - p1.first);
if (val > 0)
return 1;
if (val < 0)
return -1;
return 0;
}
// returns a value proportional to the distance
// between the point p and the line joining the
// points p1 and p2
int lineDist(iPair p1, iPair p2, iPair p)
{
return abs((p.second - p1.second) * (p2.first - p1.first) -
(p2.second - p1.second) * (p.first - p1.first));
}
// End points of line L are p1 and p2. side can have value
// 1 or -1 specifying each of the parts made by the line L
void quickHull(iPair a[], int n, iPair p1, iPair p2, int side)
{
int ind = -1;
int max_dist = 0;
// finding the point with maximum distance
// from L and also on the specified side of L.
for (int i = 0; i < n; i++)
{
int temp = lineDist(p1, p2, a[i]);
if (findSide(p1, p2, a[i]) == side && temp > max_dist)
{
ind = i;
max_dist = temp;
}
}
// If no point is found, add the end points
// of L to the convex hull.
if (ind == -1)
{
hull.insert(p1);
hull.insert(p2);
return;
}
// Recur for the two parts divided by a[ind]
quickHull(a, n, a[ind], p1, -findSide(a[ind], p1, p2));
quickHull(a, n, a[ind], p2, -findSide(a[ind], p2, p1));
}
void printHull(iPair a[], int n)
{
// a[i].second -> y-coordinate of the ith point
if (n < 3)
{
cout << "Convex hull not possible\n";
return;
}
// Finding the point with minimum and
// maximum x-coordinate
int min_x = 0, max_x = 0;
for (int i = 1; i < n; i++)
{
if (a[i].first < a[min_x].first)
min_x = i;
if (a[i].first > a[max_x].first)
max_x = i;
}
// Recursively find convex hull points on
// one side of line joining a[min_x] and
// a[max_x]
quickHull(a, n, a[min_x], a[max_x], 1);
// Recursively find convex hull points on
// other side of line joining a[min_x] and
// a[max_x]
quickHull(a, n, a[min_x], a[max_x], -1);
cout << "The points in Convex Hull are:\n";
while (!hull.empty())
{
cout << "(" << (*hull.begin()).first << ", "
<< (*hull.begin()).second << ") ";
hull.erase(hull.begin());
}
}
// Driver code
int main()
{
iPair a[] = { {0, 3}, {1, 1}, {2, 2}, {4, 4},
{0, 0}, {1, 2}, {3, 1}, {3, 3} };
int n = sizeof(a) / sizeof(a[0]);
printHull(a, n);
return 0;
}