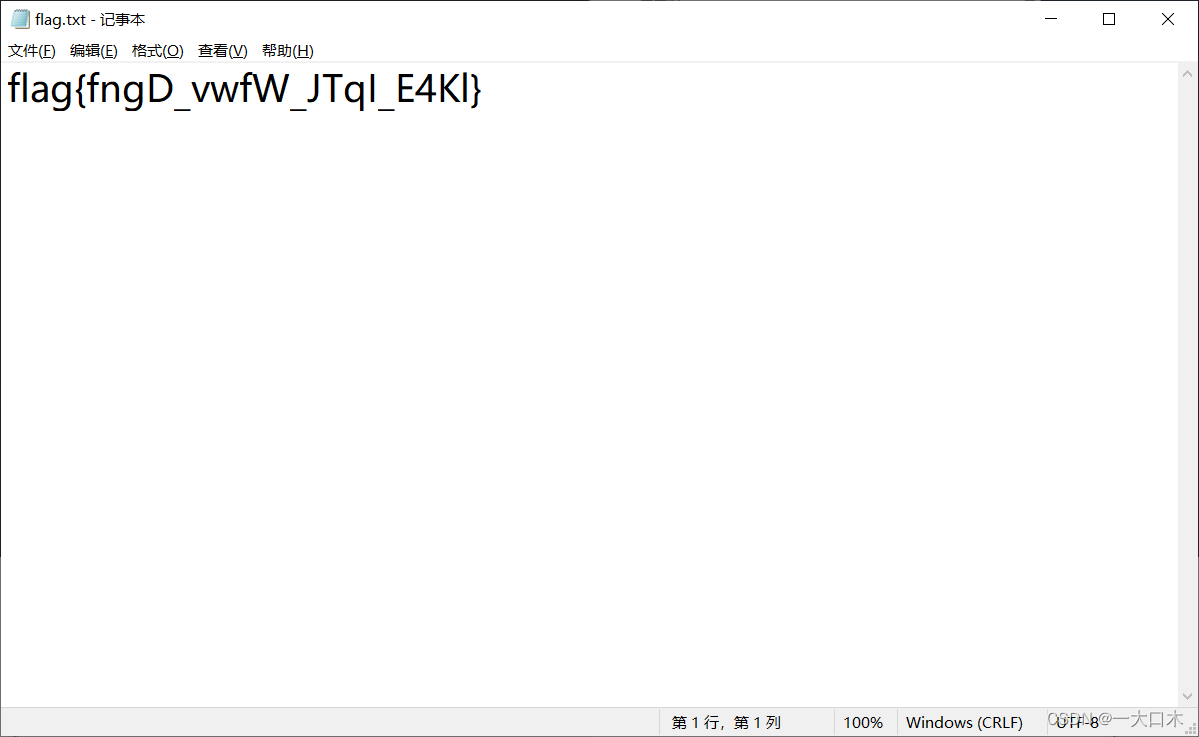
1 文本格式
using System;
using System.Text;
namespace Legalsoft.Truffer
{
/// <summary>
/// operations on polynomials
/// </summary>
public class Poly
{
/// <summary>
/// polynomial c[0]+c[1]x+c[2]x^2+ ... + c[n-2]x^n-2 + c[n-1]x^n-1
/// </summary>
private double[] c { get; set; }
/// <summary>
/// Construct polynomial
/// </summary>
/// <param name="cc"></param>
public Poly(double[] cc)
{
this.c = cc;
}
public double poly(double x)
{
int j;
double p = c[j = c.Length - 1];
while (j > 0)
{
p = p * x + c[--j];
}
return p;
}
public String toString()
{
StringBuilder sb = new StringBuilder(32);
int j = c.Length - 1;
sb.Append(String.Format("%fx^%d", c[j], j));
j--;
for (; j != 0; j--)
{
sb.Append(String.Format("%+fx^%d", c[j], j));
}
sb.Append(String.Format("%+f ", c[0]));
return sb.ToString().Substring(0);
}
/// <summary>
/// Build Polynomial from roots
/// </summary>
/// <param name="z"></param>
/// <returns></returns>
/// <exception cref="Exception"></exception>
public static Poly buildFromRoots(Complex[] z)
{
for (int i = 0; i < z.Length; i++)
{
bool found = false;
for (int j = 0; j < z.Length; j++)
{
//if (z[i].re == z[j].re && z[i].im == -z[j].im)
if (Math.Abs(z[i].re - z[j].re) <= float.Epsilon && Math.Abs(z[i].im - (-z[j].im)) <= float.Epsilon)
{
found = true;
break;
}
}
if (!found)
{
throw new Exception("Roots must be conjugate");
}
}
Complex[] c = new Complex[z.Length + 1];
c[0] = z[0].neg();
c[1] = new Complex(1, 0);
for (int i = 1; i < z.Length; i++)
{
Complex d = c[0];
c[0] = c[0].mul(z[i].neg());
for (int j = 1; j < i + 1; j++)
{
Complex dd = c[j];
c[j] = d.sub(z[i].mul(c[j]));
d = dd;
}
c[i + 1] = d;
}
double[] cc = new double[c.Length];
for (int i = 0; i < cc.Length; i++)
{
cc[i] = c[i].re;
}
return new Poly(cc);
}
/// <summary>
/// Build Polynomial from roots
/// </summary>
/// <param name="z"></param>
/// <returns></returns>
public static Poly buildFromRoots(double[] z)
{
double[] c = new double[z.Length + 1];
c[0] = -z[0]; c[1] = 1;
for (int i = 1; i < z.Length; i++)
{
double d = c[0];
c[0] *= -z[i];
for (int j = 1; j < i + 1; j++)
{
double dd = c[j];
c[j] = d - z[i] * c[j];
d = dd;
}
c[i + 1] = d;
}
return new Poly(c);
}
/// <summary>
/// Given the coefficients of a polynomial of degree nc as an array c[0..nc] of
/// size nc+1 (with c[0] being the constant term), and given a value x, this
/// routine fills an output array pd of size nd+1 with the value of the
/// polynomial evaluated at x in pd[0], and the first nd derivatives at x in
/// pd[1..nd].
/// </summary>
/// <param name="c"></param>
/// <param name="x"></param>
/// <param name="pd"></param>
public static void ddpoly(double[] c, double x, double[] pd)
{
int nc = c.Length - 1;
int nd = pd.Length - 1;
double cnst = 1.0;
pd[0] = c[nc];
for (int j = 1; j < nd + 1; j++)
{
pd[j] = 0.0;
}
for (int i = nc - 1; i >= 0; i--)
{
int nnd = (nd < (nc - i) ? nd : nc - i);
for (int j = nnd; j > 0; j--)
{
pd[j] = pd[j] * x + pd[j - 1];
}
pd[0] = pd[0] * x + c[i];
}
for (int i = 2; i < nd + 1; i++)
{
cnst *= i;
pd[i] *= cnst;
}
}
/// <summary>
/// Given the coefficients of a polynomial of degree nc as an array c[0..nc] of
/// size nc+1 (with c[0] being the constant term), and given a value x, this
/// routine fills an output array pd of size nd+1 with the value of the
/// polynomial evaluated at x in pd[0], and the first nd derivatives at x in
/// pd[1..nd].
/// </summary>
/// <param name="u"></param>
/// <param name="v"></param>
/// <param name="q"></param>
/// <param name="r"></param>
/// <exception cref="Exception"></exception>
public static void poldiv(double[] u, double[] v, double[] q, double[] r)
{
int n = u.Length - 1;
int nv = v.Length - 1;
//while (nv >= 0 && v[nv] == 0.0)
while (nv >= 0 && Math.Abs(v[nv]) <= float.Epsilon)
{
nv--;
}
if (nv < 0)
{
throw new Exception("poldiv divide by zero polynomial");
}
//r = u;
r = Globals.CopyFrom(u);
//q.assign(u.Length, 0.0);
for (int k = n - nv; k >= 0; k--)
{
q[k] = r[nv + k] / v[nv];
for (int j = nv + k - 1; j >= k; j--)
{
r[j] -= q[k] * v[j - k];
}
}
for (int j = nv; j <= n; j++)
{
r[j] = 0.0;
}
}
}
}
2 代码格式
using System;
using System.Text;
namespace Legalsoft.Truffer
{
/// <summary>
/// operations on polynomials
/// </summary>
public class Poly
{
/// <summary>
/// polynomial c[0]+c[1]x+c[2]x^2+ ... + c[n-2]x^n-2 + c[n-1]x^n-1
/// </summary>
private double[] c { get; set; }
/// <summary>
/// Construct polynomial
/// </summary>
/// <param name="cc"></param>
public Poly(double[] cc)
{
this.c = cc;
}
public double poly(double x)
{
int j;
double p = c[j = c.Length - 1];
while (j > 0)
{
p = p * x + c[--j];
}
return p;
}
public String toString()
{
StringBuilder sb = new StringBuilder(32);
int j = c.Length - 1;
sb.Append(String.Format("%fx^%d", c[j], j));
j--;
for (; j != 0; j--)
{
sb.Append(String.Format("%+fx^%d", c[j], j));
}
sb.Append(String.Format("%+f ", c[0]));
return sb.ToString().Substring(0);
}
/// <summary>
/// Build Polynomial from roots
/// </summary>
/// <param name="z"></param>
/// <returns></returns>
/// <exception cref="Exception"></exception>
public static Poly buildFromRoots(Complex[] z)
{
for (int i = 0; i < z.Length; i++)
{
bool found = false;
for (int j = 0; j < z.Length; j++)
{
//if (z[i].re == z[j].re && z[i].im == -z[j].im)
if (Math.Abs(z[i].re - z[j].re) <= float.Epsilon && Math.Abs(z[i].im - (-z[j].im)) <= float.Epsilon)
{
found = true;
break;
}
}
if (!found)
{
throw new Exception("Roots must be conjugate");
}
}
Complex[] c = new Complex[z.Length + 1];
c[0] = z[0].neg();
c[1] = new Complex(1, 0);
for (int i = 1; i < z.Length; i++)
{
Complex d = c[0];
c[0] = c[0].mul(z[i].neg());
for (int j = 1; j < i + 1; j++)
{
Complex dd = c[j];
c[j] = d.sub(z[i].mul(c[j]));
d = dd;
}
c[i + 1] = d;
}
double[] cc = new double[c.Length];
for (int i = 0; i < cc.Length; i++)
{
cc[i] = c[i].re;
}
return new Poly(cc);
}
/// <summary>
/// Build Polynomial from roots
/// </summary>
/// <param name="z"></param>
/// <returns></returns>
public static Poly buildFromRoots(double[] z)
{
double[] c = new double[z.Length + 1];
c[0] = -z[0]; c[1] = 1;
for (int i = 1; i < z.Length; i++)
{
double d = c[0];
c[0] *= -z[i];
for (int j = 1; j < i + 1; j++)
{
double dd = c[j];
c[j] = d - z[i] * c[j];
d = dd;
}
c[i + 1] = d;
}
return new Poly(c);
}
/// <summary>
/// Given the coefficients of a polynomial of degree nc as an array c[0..nc] of
/// size nc+1 (with c[0] being the constant term), and given a value x, this
/// routine fills an output array pd of size nd+1 with the value of the
/// polynomial evaluated at x in pd[0], and the first nd derivatives at x in
/// pd[1..nd].
/// </summary>
/// <param name="c"></param>
/// <param name="x"></param>
/// <param name="pd"></param>
public static void ddpoly(double[] c, double x, double[] pd)
{
int nc = c.Length - 1;
int nd = pd.Length - 1;
double cnst = 1.0;
pd[0] = c[nc];
for (int j = 1; j < nd + 1; j++)
{
pd[j] = 0.0;
}
for (int i = nc - 1; i >= 0; i--)
{
int nnd = (nd < (nc - i) ? nd : nc - i);
for (int j = nnd; j > 0; j--)
{
pd[j] = pd[j] * x + pd[j - 1];
}
pd[0] = pd[0] * x + c[i];
}
for (int i = 2; i < nd + 1; i++)
{
cnst *= i;
pd[i] *= cnst;
}
}
/// <summary>
/// Given the coefficients of a polynomial of degree nc as an array c[0..nc] of
/// size nc+1 (with c[0] being the constant term), and given a value x, this
/// routine fills an output array pd of size nd+1 with the value of the
/// polynomial evaluated at x in pd[0], and the first nd derivatives at x in
/// pd[1..nd].
/// </summary>
/// <param name="u"></param>
/// <param name="v"></param>
/// <param name="q"></param>
/// <param name="r"></param>
/// <exception cref="Exception"></exception>
public static void poldiv(double[] u, double[] v, double[] q, double[] r)
{
int n = u.Length - 1;
int nv = v.Length - 1;
//while (nv >= 0 && v[nv] == 0.0)
while (nv >= 0 && Math.Abs(v[nv]) <= float.Epsilon)
{
nv--;
}
if (nv < 0)
{
throw new Exception("poldiv divide by zero polynomial");
}
//r = u;
r = Globals.CopyFrom(u);
//q.assign(u.Length, 0.0);
for (int k = n - nv; k >= 0; k--)
{
q[k] = r[nv + k] / v[nv];
for (int j = nv + k - 1; j >= k; j--)
{
r[j] -= q[k] * v[j - k];
}
}
for (int j = nv; j <= n; j++)
{
r[j] = 0.0;
}
}
}
}