打卡记录
合法分组的最少组数(贪心)
链接
举例说明,假设 c n t [ x ] = 32 cnt[x]=32 cnt[x]=32, k = 10 k=10 k=10,那么 32 = 10 + 10 + 10 + 2 32=10+10+10+2 32=10+10+10+2,多出的 2 2 2 可以分成两个 1 1 1,加到两个 10 10 10 中,从而得到 11 , 11 , 10 11,11,10 11,11,10 三组。通过 c n t [ x ] / k cnt[x] / k cnt[x]/k 与 c n t [ x ] cnt[x] % k cnt[x] 之间的大小关系,从而来判断当前是否可以用 k k k 为最小的组元素个数来分组,如果不行的话,让 k k k 数值减少,然后再次判断。如果可以分组,则当前组 c n t [ x ] cnt[x] cnt[x] 可以分成 ⌈ c n t [ x ] k + 1 ⌉ ⌈\frac{cnt[x]}{k+1} ⌉ ⌈k+1cnt[x]⌉ 个组。
⌈ c n t [ x ] k + 1 ⌉ ⌈\frac{cnt[x]}{k+1} ⌉ ⌈k+1cnt[x]⌉ 可以由 k = 10,30…33 这样推出
class Solution {
public:
int minGroupsForValidAssignment(vector<int>& nums) {
unordered_map<int, int> hash;
for (int& num : nums) hash[num]++;
int k = min_element(hash.begin(), hash.end(), [&](const auto& a, const auto& b) {
return a.second < b.second;
})->second;
int ans = 0;
while (!ans) {
for (auto& [_, x] : hash) {
if (x / k < x % k) {
ans = 0;
break;
}
ans += (x + k) / (k + 1);
}
k--;
}
return ans;
}
};
做菜顺序(递推)
链接
从大到小排序,得到递推公式:f(k)=f(k−1)+(a[0]+a[1]+⋯+a[k−1])
例如,做 k=1,2,3 道菜时。
k=1 时,总和为 f(1)=a[0]。
k=2 时,总和为 f(2)=2⋅a[0]+a[1]。
k=3 时,总和为 f(3)=3⋅a[0]+2⋅a[1]+a[2]。
class Solution {
public:
int maxSatisfaction(vector<int>& satisfaction) {
sort(satisfaction.begin(), satisfaction.end(), greater<int>());
int n = satisfaction.size(), sum = 0, f = 0;
for (int i = 0; i < n; ++i) {
sum += satisfaction[i];
if (sum <= 0) break;
f += sum;
}
return f;
}
};
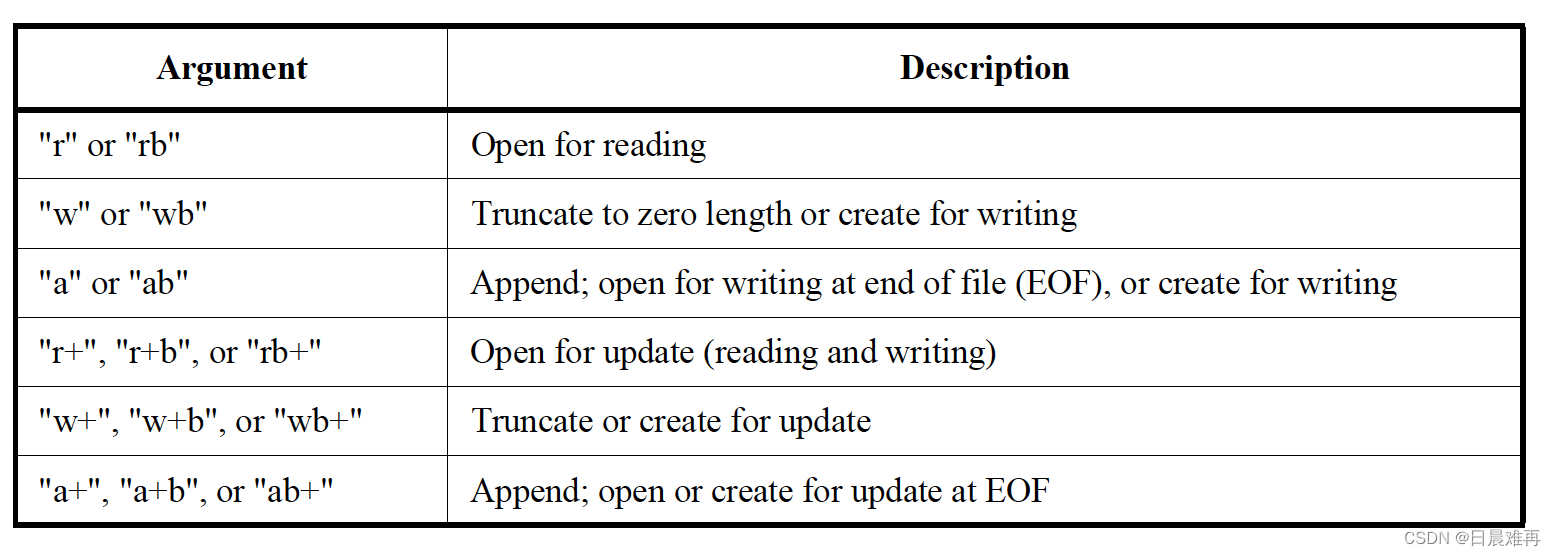


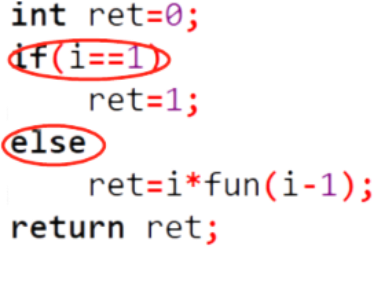

![[AUTOSAR][诊断管理][ECU][$14] 清除诊断相关信息](https://img-blog.csdnimg.cn/c30756396a6849e69486bfc85d382641.png)