🔗 运行环境：Matlab

🚩 撰写作者：左手の明天

🥇 精选专栏：《python》

🔥  推荐专栏：《算法研究》

#### 防伪水印——左手の明天 ####

💗 大家好🤗🤗🤗，我是左手の明天！好久不见💗

💗今天开启新的系列——重新定义matlab强大系列💗

📆  最近更新：2023 年 10 月 14 日，左手の明天的第 293 篇原创博客

📚 更新于专栏：matlab

#### 防伪水印——左手の明天 ####

---

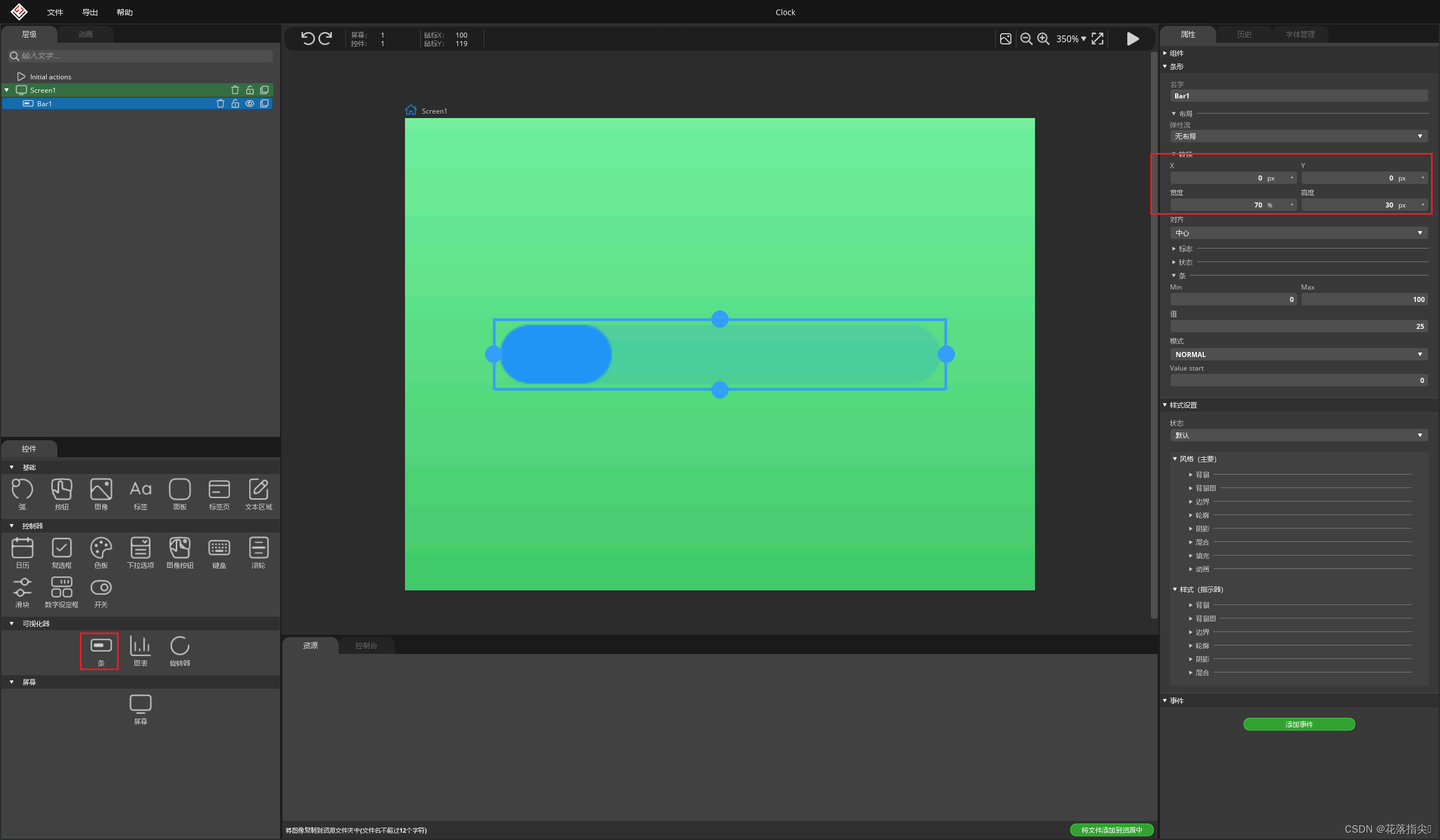
问题描述

要混合具有不同化学组成的钢材，以获得 25 吨具有某一特定化学组成的钢材。所得钢材应包含 5% 的碳和 5% 的钼（以重量计），即 25 吨 *5% = 1.25 吨碳和 1.25 吨钼。目标是将混合钢材的成本降至最低。

有四种钢锭可供购买。每种钢锭只能购买一块。

有三种等级的合金钢和一种等级的废钢可供购买。合金和废钢不必整吨购买。

表示问题

要表示此问题，首先要确定控制项变量。以变量 ingots(1) = 1 表示您购买钢锭 1，ingots(1) = 0 表示您不购买此钢锭。类似地，变量 ingots(2) 至 ingots(4) 也是二元变量，用于指示您是否购买钢锭 2 至 4。

变量 alloys(1) 至 alloys(3) 分别是您购买的合金 1、2 和 3 的吨数，scrap 是您购买的废钢的吨数。

steelprob = optimproblem;
ingots = optimvar('ingots',4,'Type','integer','LowerBound',0,'UpperBound',1);
alloys = optimvar('alloys',3,'LowerBound',0);
scrap = optimvar('scrap','LowerBound',0);

创建与变量相关联的成本表达式。

weightIngots = [5,3,4,6];
costIngots = weightIngots.*[350,330,310,280];
costAlloys = [500,450,400];
costScrap = 100;
cost = costIngots*ingots + costAlloys*alloys + costScrap*scrap;

将成本作为目标函数包含在问题中。

steelprob.Objective = cost;

该问题有三个等式约束。第一个约束是总重量为 25 吨。计算钢的重量。

totalWeight = weightIngots*ingots + sum(alloys) + scrap;

第二个约束是碳的重量为 25 吨的 5%，即 1.25 吨。计算钢中碳的重量。

carbonIngots = [5,4,5,3]/100;
carbonAlloys = [8,7,6]/100;
carbonScrap = 3/100;
totalCarbon = (weightIngots.*carbonIngots)*ingots + carbonAlloys*alloys + carbonScrap*scrap;

第三个约束是钼的重量为 1.25 吨。计算钢中钼的重量。

molybIngots = [3,3,4,4]/100;
molybAlloys = [6,7,8]/100;
molybScrap = 9/100;
totalMolyb = (weightIngots.*molybIngots)*ingots + molybAlloys*alloys + molybScrap*scrap;

在问题中包含约束。

steelprob.Constraints.conswt = totalWeight == 25;
steelprob.Constraints.conscarb = totalCarbon == 1.25;
steelprob.Constraints.consmolyb = totalMolyb == 1.25;

求解问题

现已具备所有输入，请调用求解器。

[sol,fval] = solve(steelprob);

Solving problem using intlinprog.
LP:                Optimal objective value is 8125.600000.                                          

Cut Generation:    Applied 3 mir cuts.                                                              
                   Lower bound is 8495.000000.                                                      
                   Relative gap is 0.00%.                                                          


Optimal solution found.

Intlinprog stopped at the root node because the objective value is within a gap tolerance of the optimal value, options.AbsoluteGapTolerance = 0. The intcon variables are integer within tolerance, options.IntegerTolerance = 1e-05.

查看解。

sol.ingots

ans = 4×1

     1
     1
     0
     1

sol.alloys

ans = 3×1

    7.0000
    0.5000
         0

sol.scrap

ans = 3.5000

fval

fval = 8495

最终结论

最优购买成本为 8495 美元。购买钢锭 1、2 和 4，但不购买 3，并购买 7.25 吨合金 1、0.25 吨合金 3 和 3.5 吨废钢。