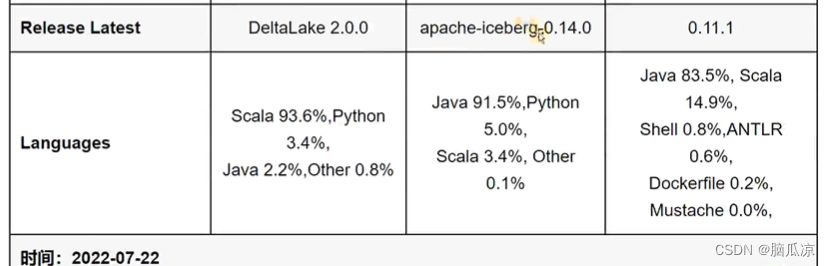
https://www.luogu.com.cn/problem/CF1592F1

场上猜了个结论，感觉只会操作1。然后被样例1hack了。然后就猜如果 $(n,m)$ 为1则翻转4操作，被#14hack了。然后就猜4操作只会进行一次，然后就不知道怎么做下去了。

---

上面猜的结论都正确，但是既然猜结论了，为什么不考虑先证明一波？

考虑2次操作4，代价为6，只有两种情况：

而他们都可以用操作1表示出来。

然后考虑怎么做。其实感觉没有操作4时，每个位置是否翻转都可以直接O（1）算出来。但这存在一定难度。

我当时写的是：

这样子存在逻辑联系，不方便直接表示，所以应该考虑把if取得。

怎么去？就多列几个表示出来。（相当于多一个媒介）

$$\begin{gathered} v_{i,j}=a_{i,j}\otimes s_{i-1,j}\otimes s_{i,j-1}\otimes s_{i-1,j-1} \\ {p}_{i,j}=v_{i,j}\otimes s_{i-1,j}\otimes s_{i,j-1}\otimes s_{i-1,j-1} \end{gathered}$$

然后我们发现了 $s$ 和 $a$ 相同。

然后发现翻转4只会改变4个位置。

然后操作4有贡献只当这4个位置同时改变。

#include<bits/stdc++.h>
using namespace std;
//#define int long long
inline int read(){int x=0,f=1;char ch=getchar();
while(ch<'0'||ch>'9'){if(ch=='-')f=-1;
ch=getchar();}while(ch>='0'&&ch<='9'){x=(x<<1)+
(x<<3)+(ch^48);ch=getchar();}return x*f;}
#define Z(x) (x)*(x)
#define pb push_back
//#define M
//#define mo
#define N 510
int n, m, i, j, k, T;
int a[N][N], p[N][N], ans; 
char str[N]; 

signed main()
{
//	freopen("in.txt", "r", stdin);
//	freopen("out.txt", "w", stdout);
//	srand(time(NULL));
//	T=read();
//	while(T--) {
//
//	}
	auto calc = [&] (int x, int y) -> int {
		return a[x][y]^a[x+1][y]^a[x][y+1]^a[x+1][y+1]; 
	}; 
	n=read(); m=read(); 
	for(i=1; i<=n; ++i) {
		scanf("%s", str+1); 
		for(j=1; j<=m; ++j) 
			if(str[j]=='B') a[i][j]=1; 
	}
	for(i=n; i>=1; --i) 	
		for(j=m; j>=1; --j) {
			p[i][j]=(p[i+1][j]^p[i][j+1]^p[i+1][j+1]); 
			if(a[i][j]^p[i][j]) p[i][j]^=1, ++ans; 
			ans+=calc(i, j); 
			if(i!=n && j!=m && calc(i, j) && calc(i, m) && calc(n, j) && calc(n, m)) k=-1; 
		}

	printf("%d", ans+k); 
	return 0;
}