在这里插入图片描述

快速排序详解

快速排序
- 1. 挖坑法
- 2. 左右指针法（Hoare 法）
- 3. 前后指针法
- 4. 快排非递归
代码优化

排序：排序，就是使一串记录，按照其中的某个或某些关键字的大小，递增或递减的排列起来的操作。

稳定性：假定在待排序的记录序列中，存在多个具有相同的关键字的记录，若经过排序，这些记录的相对次序保持不变，即在原序列中， r[i] = r[j]，且 r[i] 在 r[j] 之前，而在排序后的序列中， r[i] 仍在 r[j] 之前，则称这种排序算法是稳定的；否则称为不稳定的。
（注意稳定排序可以实现为不稳定的形式，而不稳定的排序实现不了稳定的形式）

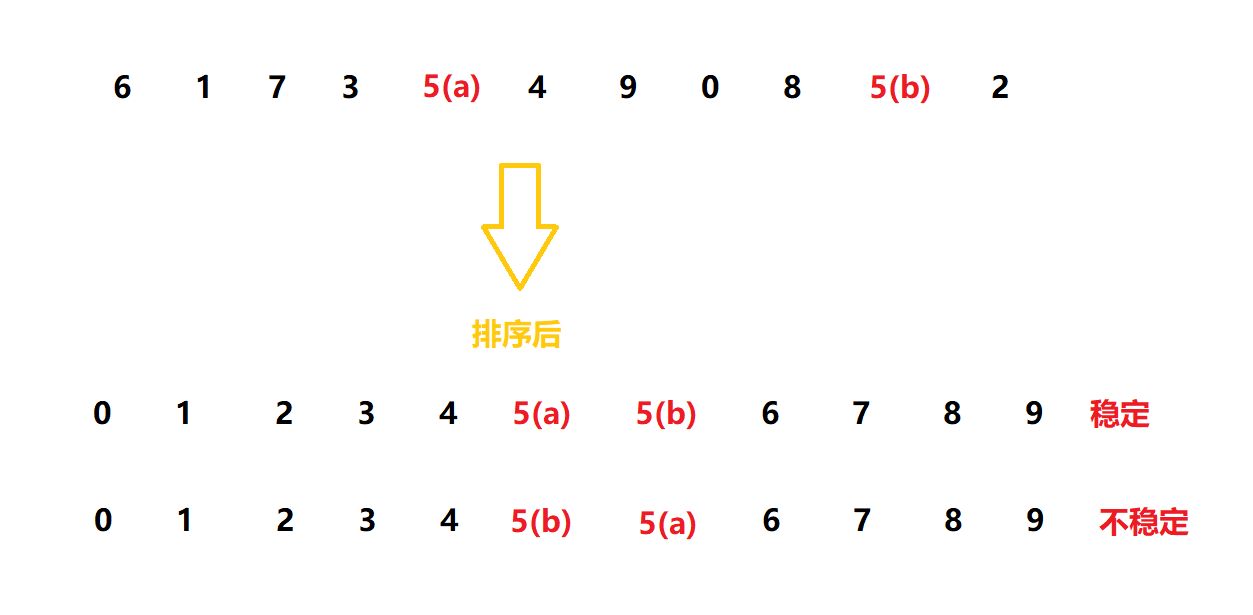
在这里插入图片描述

内部排序：数据元素全部放在内存中的排序。

外部排序：数据元素太多不能同时放在内存中，根据排序过程的要求不能在内外存之间移动数据的排序。

快速排序

快速排序是 Hoare 于 1962 年提出的一种二叉树结构的交换排序方法，其基本思想为：任取待排序元素序列中的某元素作为基准值，按照该排序码将待排序集合分割成两子序列，左子序列中所有元素均小于基准值，右子序列中所有元素均大于基准值，然后最左右子序列重复该过程，直到所有元素都排列在相应位置上为止。

1. 挖坑法

选择基准元素：从待排序的数组中选择一个元素作为基准（pivot）。通常情况下，可以选择第一个元素、最后一个元素、中间元素或者随机选择一个元素作为基准。
分割数组：从数组的两端开始，分别设置两个指针，一个从左边（low指针）开始，一个从右边（high指针）开始，分别向中间移动，直到它们相遇。在移动过程中，通过比较元素与基准的大小关系，找到一个大于基准的元素和一个小于基准的元素，并将它们互换位置。
继续分割：重复步骤2，直到low指针和high指针相遇。在此过程中，将小于基准的元素移到基准的左侧，将大于基准的元素移到基准的右侧，形成三个区域。
递归排序：对左侧小于基准的区域和右侧大于基准的区域，分别递归地应用快速排序算法。

在这里插入图片描述

    public static void quickSort(int[] arr) {
        int len = arr.length;
        partition(arr, 0, len-1);
    }

    public static void partition(int[] arr, int left, int right) {
        if (left >= right) {
            return;
        }
        int pivot = left;
        int value = arr[left];
        // 从两边开始遍历
        int begin = left;
        int end = right;
        while (begin < end) {
            // 注意一定要带上 ==, 不然死循环
            while (begin < end && arr[end] >= value) {
                end--;
            }
            // 右边找到小于 value 的值
            arr[pivot] = arr[end];
            // end 变为 坑
            pivot = end;
            while (begin < end && arr[begin] <= value) {
                begin++;
            }
            // 左边找到大于 value 的值
            arr[pivot] = arr[begin];
            // begin 变为坑
            pivot = begin;
        }
        // value 找到自己的正确位置
        arr[pivot] = value;
        // 递归左边和右边
        partition(arr, left, pivot-1);
        partition(arr, pivot+1, right);
    }

2. 左右指针法（Hoare 法）

从两边开始，左边找到比基准值大的，右边找比基准值小的，找到后，两边交换，一直到左右两个指针相遇，相遇位置即为基准值的正确位置，然后递归确定左右两边的区间元素的位置。

	public static void quickSort(int[] arr) {
        int len = arr.length;
        partition(arr, 0, len-1);
    }


    public static void partition(int[] arr, int left, int right) {
        if (left >= right) {
            return;
        }
        int value = arr[left];
        int begin = left;
        int end = right;
        while (begin < end) {
            // 注意一定要带上 ==, 不然死循环
            while (begin < end && arr[end] >= value) {
                end--;
            }
            while (begin < end && arr[begin] <= value) {
                begin++;
            }
            swap(arr, begin, end);
        }
        swap(arr, left, begin);
        partition(arr, left, begin-1);
        partition(arr, begin+1, right);
    }
    public static void swap (int[] arr, int index1, int index2) {
        int temp = arr[index1];
        arr[index1] = arr[index2];
        arr[index2] = temp;
    }

3. 前后指针法

后面的指针指向小于基准值的最后一个，前面的指针一直往后找，找到小于基准值的就与后面指针的下一个位置交换，后面的指针 ++，直到前面的指针遍历完，最后后面的指针的位置，就是基准值的正确位置。然后再递归确定左右区间的元素的位置。

    public static void quickSort(int[] arr) {
        int len = arr.length;
        partition(arr, 0, len-1);
    }
    
    public static void partition(int[] arr, int left, int right) {
        if (left >= right) {
            return;
        }
        int value = arr[left];
        // 前后指针
        int end = left;
        int front = left + 1;
        while (front <= right) {
            if (arr[front] < value) {
                swap(arr, end+1, front);
                end++;
            }
            front++;
        }
        swap(arr, left, end);
        // 递归左边和右边
        partition(arr, left, end-1);
        partition(arr, end+1, right);
    }
    
    public static void swap (int[] arr, int index1, int index2) {
        int temp = arr[index1];
        arr[index1] = arr[index2];
        arr[index2] = temp;
    }

4. 快排非递归

使用栈记录要排序的区间

    public static void quickSortNonR(int[] arr) {
        int len = arr.length;
        Stack<Integer> stack = new Stack<>();
        stack.push(arr.length-1);
        stack.push(0);
        while (!stack.isEmpty()) {
            int left = stack.pop();
            int right = stack.pop();

            if (left >= right) {
                continue;
            }
            int pivot = left;
            int value = arr[left];
            // 从两边开始遍历
            int begin = left;
            int end = right;
            while (begin < end) {
                // 注意一定要带上 ==, 不然死循环
                while (begin < end && arr[end] >= value) {
                    end--;
                }
                // 右边找到小于 value 的值
                arr[pivot] = arr[end];
                // end 变为 坑
                pivot = end;
                while (begin < end && arr[begin] <= value) {
                    begin++;
                }
                // 左边找到大于 value 的值
                arr[pivot] = arr[begin];
                // begin 变为坑
                pivot = begin;
            }
            // value 找到自己的正确位置
            arr[pivot] = value;
            // 右区间
            stack.push(right);
            stack.push(pivot+1);
            // 左区间
            stack.push(pivot-1);
            stack.push(left);
        }
    }

代码优化

优化一：
三数取中：
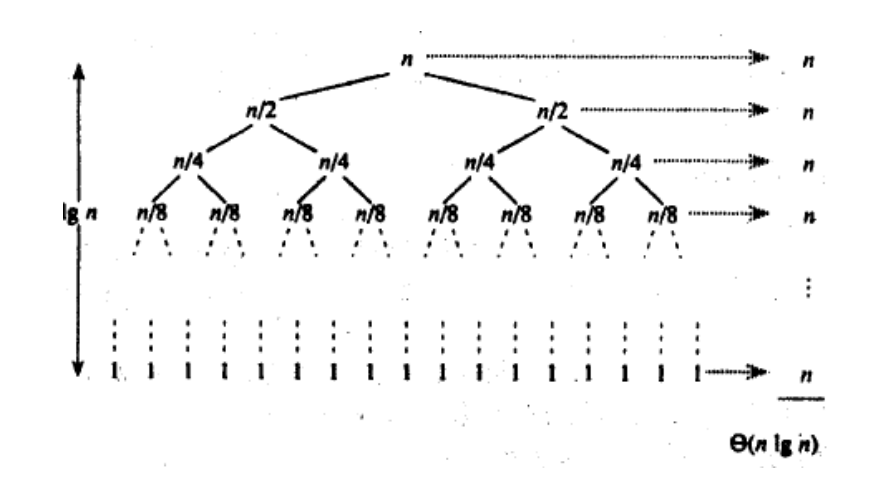
当我们找基准值时，基准值越靠近是中位数，每次都近似于将一个大的区间分成两个相等的区间，那么时间复杂度越低，越靠近是 O（N*logN）, 最坏的情况下，每次确定一个元素，即分成的两个区间其中一个只有一个元素，那么如果每次都是这样的话，最终的时间复杂度为 O（N*N），所以选择基准值时，越靠近中位数越好。

这里面以前后指针为例使用了三数取中



    public static void quickSort(int[] arr) {
        int len = arr.length;
        partition(arr, 0, len-1);
    }

    /**
     *  前后指针
     */

    public static void partition(int[] arr, int left, int right) {
        if (left >= right) {
            return;
        }
        // 三数取中
        int midIndex = getMidIndex(arr, left, right);
        swap(arr, left, midIndex);

        int value = arr[left];
        // 前后指针
        int end = left;
        int front = left + 1;
        while (front <= right) {
            if (arr[front] < value) {
                swap(arr, end+1, front);
                end++;
            }
            front++;
        }
        swap(arr, left, end);
        // 递归左边和右边
        partition(arr, left, end-1);
        partition(arr, end+1, right);
    }



    public static int getMidIndex(int[] arr, int left, int right) {
        int midIndex = ((right - left) >> 1) + left;
        if (arr[left] <= arr[midIndex]) {
            if (arr[midIndex] <= arr[right]) {
                return midIndex;
            } else {
                if (arr[left] >= arr[right]) {
                    return left;
                } else {
                    return right;
                }
            }
        } else {
            if (arr[midIndex] >= arr[right]) {
                return midIndex;
            } else {
                if (arr[left] >= arr[right]) {
                    return right;
                } else {
                    return left;
                }
            }
        }
    }



    public static void swap (int[] arr, int index1, int index2) {
        int temp = arr[index1];
        arr[index1] = arr[index2];
        arr[index2] = temp;
    }

优化二：
小区间优化，当区间中元素数量比较少时，区间中元素本身就接近有序，使用直接插入排序能提高效率（直接插入排序）
假如说有 100W 个数据，就会调用 100W 次 partition 函数，但是光最后两层就会调用近 80W 次，所以使用小区间优化提高效率。

直接插入排序

    public static void insertSort(int[] arr) {
        int len = arr.length;
        for (int i = 1; i < len; i++) {
            // 从已经有序的位置从后往前开始比较
            int key = arr[i];
            int end = i-1;
            while (end >= 0 && arr[end] > key) {
                // 数据往后挪
                arr[end+1] = arr[end];
                end--;
            }
            // 找到了合适位置, 就插入进去
            arr[end+1] = key;
        }
    }

在快排中使用小区间优化


	 /**
     *   直接插入排序
     */
    public static void insertSort(int[] arr, int left, int right) {
        for (int i = left+1; i <= right; i++) {
            // 从已经有序的位置从后往前开始比较
            int key = arr[i];
            int end = i-1;
            while (end >= 0 && arr[end] > key) {
                // 数据往后挪
                arr[end+1] = arr[end];
                end--;
            }
            // 找到了合适位置, 就插入进去
            arr[end+1] = key;
        }
    }
	

	
    public static void quickSort(int[] arr) {
        int len = arr.length;
        partition(arr, 0, len-1);
    }

	public static void partition(int[] arr, int left, int right) {
        if (left >= right) {
            return;
        }
        // 小区间优化, 当数组中元素个数 <= 100 个时使用直接插入排序
        // 主要是减少了递归的次数
        if (right - left + 1 <= 100) {
            insertSort(arr, left, right);
            return;
        }
        // 三数取中
        int midIndex = getMidIndex(arr, left, right);
        swap(arr, left, midIndex);

        int value = arr[left];
        // 前后指针
        int end = left;
        int front = left + 1;
        while (front <= right) {
            if (arr[front] < value) {
                swap(arr, end+1, front);
                end++;
            }
            front++;
        }
        swap(arr, left, end);
        // 递归左边和右边
        partition(arr, left, end-1);
        partition(arr, end+1, right);
    }