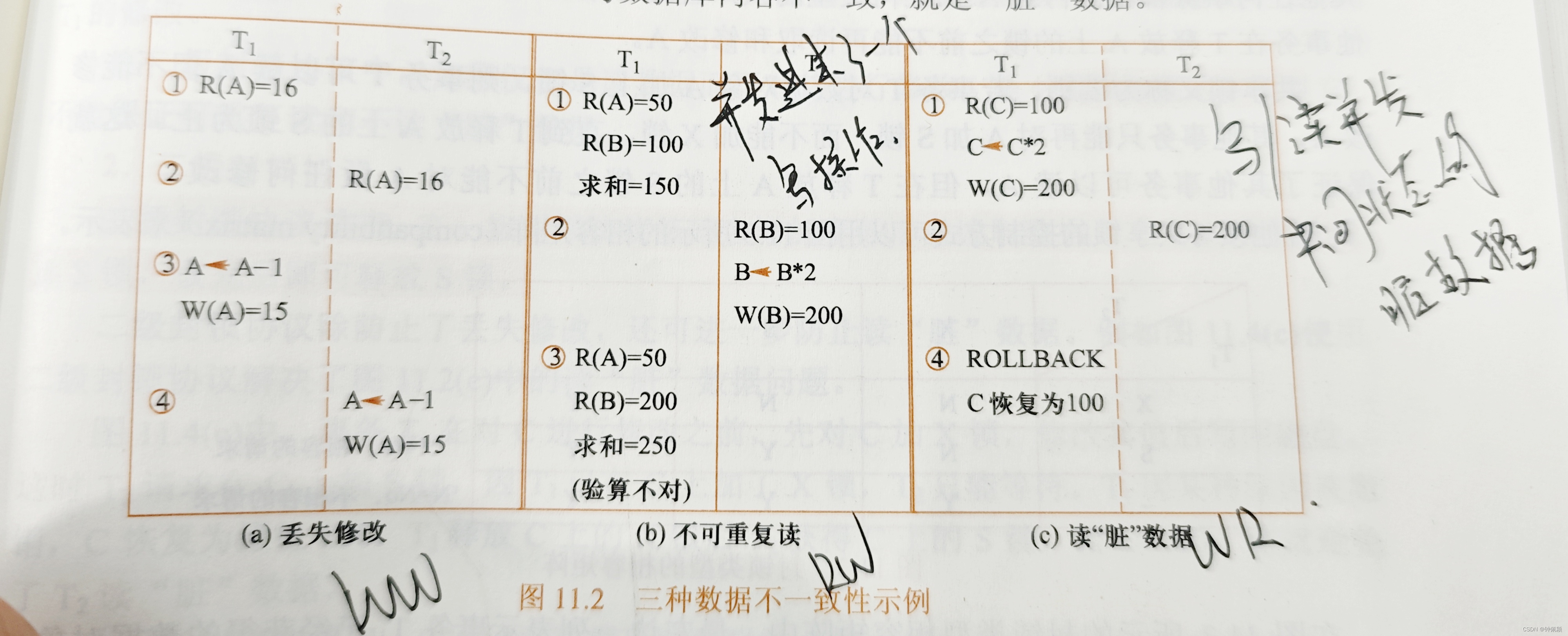
算法思想

深度优先搜索（DFS）：

• 使用递归从节点 0 开始，探索所有从当前节点到终点 n−1 的路径。
• 每次访问一个节点时，将该节点加入当前路径 path。

回溯法： 在递归返回时，通过 path.pop_back() 撤销当前节点的选择，回到上一层状态，避免影响其他分支。
路径保存： 当递归到达终点 n−1 时，将当前路径加入结果集 result。
递归终止条件： 当前节点为终点 n−1 时，结束递归。
复杂度： 针对有向无环图（DAG）的特性，确保路径无环，且不重复访问节点。

#include <iostream>
#include <vector>

using namespace std;

class Solution {
public:
    vector<vector<int>> allPathsSourceTarget(vector<vector<int>>& graph) {
        vector<vector<int>> result;  // 存储所有路径
        vector<int> path;            // 当前路径
        dfs(graph, 0, path, result); // 从节点0开始搜索
        return result;
    }

private:
    void dfs(vector<vector<int>>& graph, int node, vector<int>& path, vector<vector<int>>& result) {
        path.push_back(node); // 将当前节点加入路径
        if (node == graph.size() - 1) {
            // 如果到达目标节点，将路径加入结果
            result.push_back(path);
        }
        else {
            // 遍历当前节点的所有邻居
            for (int neighbor : graph[node]) {
                dfs(graph, neighbor, path, result);
            }
        }
        path.pop_back(); // 回溯，移除当前节点
    }
};

int main() {
    // 输入图
    vector<vector<int>> graph = { {1, 2}, {3}, {3}, {} };
    Solution solution;
    vector<vector<int>> result = solution.allPathsSourceTarget(graph);

    // 输出结果
    cout << "[" << endl;
    for (const auto& path : result) {
        cout << "  [";
        for (int i = 0; i < path.size(); ++i) {
            cout << path[i];
            if (i != path.size() - 1) cout << ", ";
        }
        cout << "]" << endl;
    }
    cout << "]" << endl;

    return 0;
}