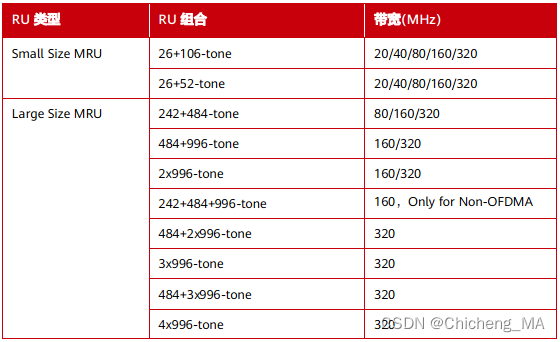
例一 空气被充入一个球形气球中，使其体积以每秒100立方厘米的速度增加。当气球的直径为50厘米时，气球半径的增加速度是多少？

解答：球体的体积公式为
$$V=\frac{4}{3}\pi r^3$$

为了使用给定的信息，我们对该方程的两边关于 $t$ 求导。为了对右侧求导，我们需要使用链式法则：

$$\frac{dV}{dt}=\frac{dV}{dr}\cdot \frac{dr}{dt}=4\pi r^2\cdot \frac{dr}{dt}$$

现在我们求未知量：

$$\frac{dr}{dt}=\frac{1}{4\pi r^2}\cdot \frac{dV}{dt}$$

如果在该方程中代入 $r=25$ 和 $\frac{dV}{dt}=100$，我们得到：

$$\frac{dr}{dt}=\frac{1}{4\pi (25{)}^2}\cdot 100=\frac{1}{25\pi }$$

例二 一把10英尺长的梯子靠在垂直墙上。如果梯子的底部以每秒1英尺的速度远离墙壁，那么当梯子的底部距离墙壁6英尺时，梯子的顶部以多快的速度沿墙滑下？

解答：我们首先绘制一个图。设$x$英尺是梯子底部到墙的距离，$y$英尺是梯子顶部到地面的距离。注意，$x$和$y$都是时间$t$的函数（时间以秒为单位）。

在这个问题中，$x$和$y$之间的关系由勾股定理给出：

$$x^2+y^2=100$$

对每一侧使用链式法则对(t)进行求导，我们得到

$$2x\frac{dx}{dt}+2y\frac{dy}{dt}=0$$

解这个方程得到所需的速度：

$$\frac{dy}{dt}=-\frac{x}{y}\frac{dx}{dt}$$

当$x=6$时，由勾股定理可得$y=8$，因此，代入这些数值$\frac{dx}{dt}=1$，我们得到

$$\frac{dy}{dt}=-\frac{6}{8}(1)=-\frac{3}{4}\text{ 英尺/秒}$$

例三 一个水箱的形状是倒置的圆锥形，底面半径为2米，高为4米。如果水以每分钟2立方米的速度注入水箱，求当水深为3米时水位上升的速度。

解答：我们首先绘制圆锥并如图标记。设 $V$、$r$ 和 $h$ 分别为水的体积、表面半径和水的高度，时间 $t$ 以分钟为单位。

我们知道 $\frac{dV}{dt}=2\text{ 立方米/分钟}$，要求当 $h=3$ 米时的 $\frac{dh}{dt}$。$V$ 和 $h$ 的关系由以下公式给出：

$$V=\frac{1}{3}\pi r^{2}h$$

但将 $V$ 表示为仅关于 $h$ 的函数更为有用。为了消去 $r$，我们使用图3中的相似三角形写出：

$$\frac{r}{h}=\frac{2}{4}⟹r=\frac{h}{2}$$

则 $V$ 的表达式变为：

$$V=\frac{1}{3}\pi {\left(\frac{h}{2}\right)}^{2}h=\frac{\pi }{12}{h}^3$$

现在我们可以对每一侧关于 $t$ 进行求导：

$$\frac{dV}{dt}=\frac{\pi }{4}{h}^2\frac{dh}{dt}$$

因此

$$\frac{dh}{dt}=\frac{4}{\pi h^2}\frac{dV}{dt}$$

代入 $h=3$ 米和 $\frac{dV}{dt}=2\text{ 立方米/分钟}$，我们得到：

$$\frac{dh}{dt}=\frac{4}{\pi (3{)}^2}\cdot 2=\frac{8}{9\pi }$$

水位上升的速度为 $\frac{8}{9\pi }\approx 0.28\text{ 米/分钟}$。

例四 A车以每小时50英里的速度向西行驶，B车以每小时60英里的速度向北行驶。两车都驶向两条路的交叉口。当A车距离交叉口0.3英里，B车距离交叉口0.4英里时，两车以多快的速度相互接近？

解答：我们绘制图，其中 $C$ 是道路的交叉口。在给定的时间 $t$，设 $x$ 为A车到 $C$ 的距离，$y$ 为B车到 $C$ 的距离，$z$ 为两车之间的距离，$x$、$y$ 和 $z$ 以英里为单位。

我们知道 $\frac{dx}{dt}=-50$ 英里/小时和 $\frac{dy}{dt}=-60$ 英里/小时（导数为负数，因为 $x$ 和 $y$ 随着 $t$ 的增加而减少）。我们要求 $\frac{dz}{dt}$。 $x$、$y$ 和 $z$ 之间的关系由勾股定理给出：

$$z^2=x^2+y^2$$

对每一侧关于 $t$ 进行求导，我们得到

$$2z\frac{dz}{dt}=2x\frac{dx}{dt}+2y\frac{dy}{dt}$$

$$\frac{dz}{dt}=\frac{1}{z}\left(x\frac{dx}{dt}+y\frac{dy}{dt}\right)$$

当 $x=0.3$ 英里和 $y=0.4$ 英里时，勾股定理得出 $z=0.5$ 英里，因此

$$\frac{dz}{dt}=\frac{1}{0.5}\left[0.3(-50)+0.4(-60)\right]=-78\text{ 英里/小时}$$

两车相互接近的速度为每小时78英里。

例五 一个人沿着直路以每秒4英尺的速度行走。一个探照灯位于距这条路20英尺的地面上，并始终聚焦在这个人身上。当这个人距探照灯最近点15英尺时，探照灯以多快的速度旋转？

解答：我们绘制图，并设 $x$ 为人到路径上离探照灯最近点的距离。我们设 $\theta$ 为探照灯光束与路径垂线之间的角度。

我们知道 $\frac{dx}{dt}=4\text{英尺/秒}$，要求当 $x=15$ 时的 $\frac{d\theta }{dt}$。从图5可以写出 $x$ 和 $\theta$ 之间的关系：

$$\frac{x}{20}=\tan \theta ⟹x=20\tan \theta$$

对每一侧关于 $t$ 进行求导，我们得到

$$\frac{dx}{dt}=20{\sec }^2\theta \frac{d\theta }{dt}$$

因此

$$\frac{d\theta }{dt}=\frac{\frac{dx}{dt}}{20{\sec }^2\theta }$$

当 $x=15$ 时，

$$15=20\tan \theta ⟹\tan \theta =\frac{15}{20}=\frac{3}{4}$$

因此

$$\sec \theta =\sqrt{1+{\tan }^2\theta }=\sqrt{1+{\left(\frac{3}{4}\right)}^2}=\sqrt{1+\frac{9}{16}}=\sqrt{\frac{25}{16}}=\frac{5}{4}$$

代入 $\frac{dx}{dt}=4$ 和 $\sec \theta =\frac{5}{4}$，我们得到：

$$\frac{d\theta }{dt}=\frac{4}{20{\left(\frac{5}{4}\right)}^2}=\frac{4}{20\cdot \frac{25}{16}}=\frac{4\cdot 16}{20\cdot 25}=\frac{64}{500}=\frac{16}{125}\approx 0.128\text{弧度/秒}$$

探照灯旋转的速度约为每秒0.128弧度。