文章目录
- 前言
- 104.二叉树的最大深度
- 思路
- 知识点
- 方法一 递归法
- 方法二 迭代法
- 559. n叉树的最大深度
- 111.二叉树的最小深度
- 思路
- 方法一 后向遍历递归法
- 方法二 迭代法
- 222.完全二叉树的节点个数
- 思路
- 方法一 当成普通二叉树来做
- 方法二 利用完全二叉树的特性
- 总结
前言
所有的题目一刷都是优先掌握递归,迭代法没看,记不住。打十个做完之后再说吧
104和111没有看先序遍历的代码
104.二叉树的最大深度
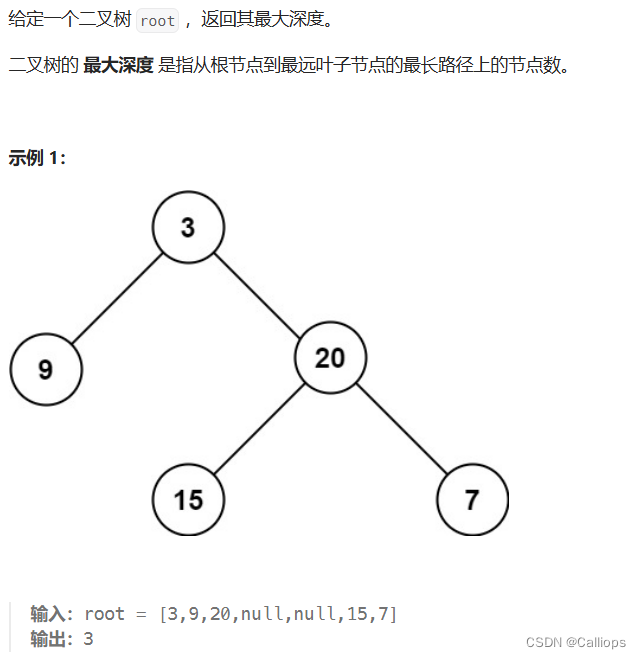
思路
知识点
记住深度和高度的定义:1. 从1开始 计数 2. 深度和高度与我们主观常识一致


总体思路:求解最大深度就是求解根节点的高度;💛
因为求深度是前序遍历,求高度是后序遍历【在子节点的高度上加1就是根节点的高度】,后序遍历要比前序遍历在本题中简洁一些,所以本题使用后序遍历的求高度;
💟具体实现细节:单层递归中求解的逻辑是,当前节点的高度为子节点高度+1;【递归法的第三步】
递归三部曲
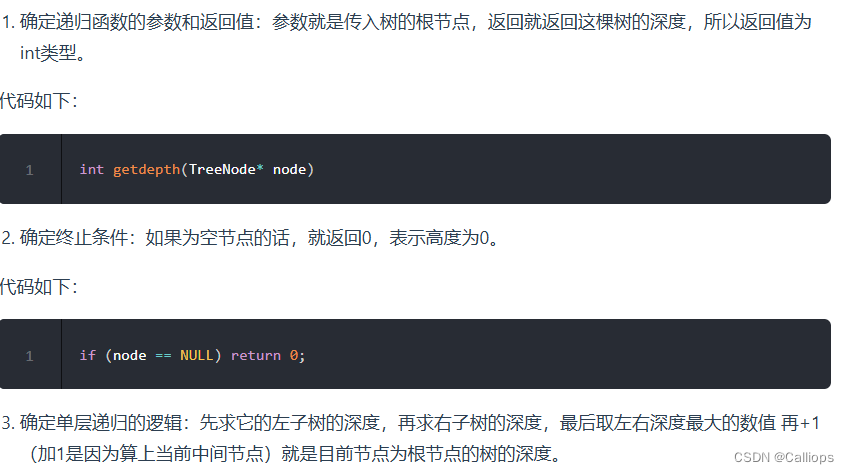
先序遍历有c++代码,非常直观的从上往下的递归。也可以看
方法一 递归法
class Solution(object):
def maxDepth(self, root):
"""
:type root: TreeNode
:rtype: int
"""
def getheight(root):
if not root: return 0
height_left = getheight(root.left)
height_right =getheight(root.right)
height = 1+max(height_left,height_right)
return height
return getheight(root)
###精简版
class Solution(object):
def maxDepth(self, root):
"""
:type root: TreeNode
:rtype: int
"""
if not root: return 0
return 1 + max(self.maxDepth(root.left), self.maxDepth(root.right))
方法二 迭代法
559. n叉树的最大深度
这题目我也刷了
class Solution:
def maxDepth(self, root: 'Node') -> int:
if not root:
return 0
max_depth = 1
for child in root.children:
max_depth = max(max_depth, self.maxDepth(child) + 1)
return max_depth
111.二叉树的最小深度
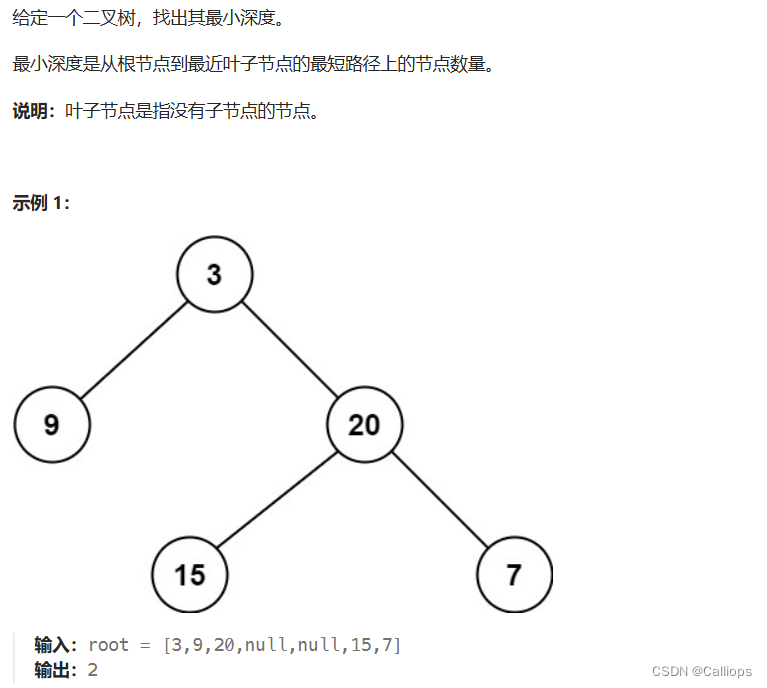
思路
总体思路:与上面的最大深度差不多,但是不能单纯将max改成min。
-
如果直接max改成min的话,例如下面的最右边的节点6会被算成高度为1,因为min子节点的结果为0,也就是将6当成叶子了;或者按照老师讲解的,根节点中会算左边的null为0.,这样最小深度就是1了。
下面是老师的讲解
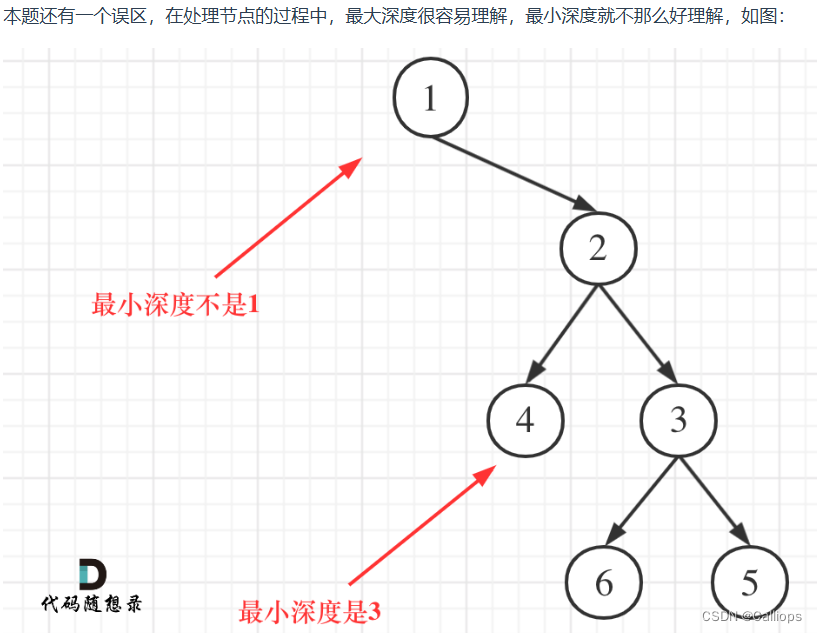
-
所以这条题目相较于104的改动就是加上分类讨论:(这是递归法的第三步单层逻辑)
- 子节点中,一个是null,一个有节点,按照有节点的那个算
- 两个都有的话选择min的那个
- 两个都是空那就是0【可以与上面那个合并】

方法一 后向遍历递归法
自己写的错误
- 在类里面调用递归的时候,记得加上self
- 下面是教程里面的代码,我写的时候is None用not来代替了,我觉着这样国家好一些
class Solution:
def getDepth(self, node):
if node is None:
return 0
leftDepth = self.getDepth(node.left) # 左
rightDepth = self.getDepth(node.right) # 右
# 当一个左子树为空,右不为空,这时并不是最低点
if node.left is None and node.right is not None:
return 1 + rightDepth
# 当一个右子树为空,左不为空,这时并不是最低点
if node.left is not None and node.right is None:
return 1 + leftDepth
result = 1 + min(leftDepth, rightDepth)
return result
def minDepth(self, root):
return self.getDepth(root)
### 精简代码
class Solution:
def minDepth(self, root):
if root is None:
return 0
if root.left is None and root.right is not None:
return 1 + self.minDepth(root.right)
if root.left is not None and root.right is None:
return 1 + self.minDepth(root.left)
return 1 + min(self.minDepth(root.left), self.minDepth(root.right))
方法二 迭代法
222.完全二叉树的节点个数
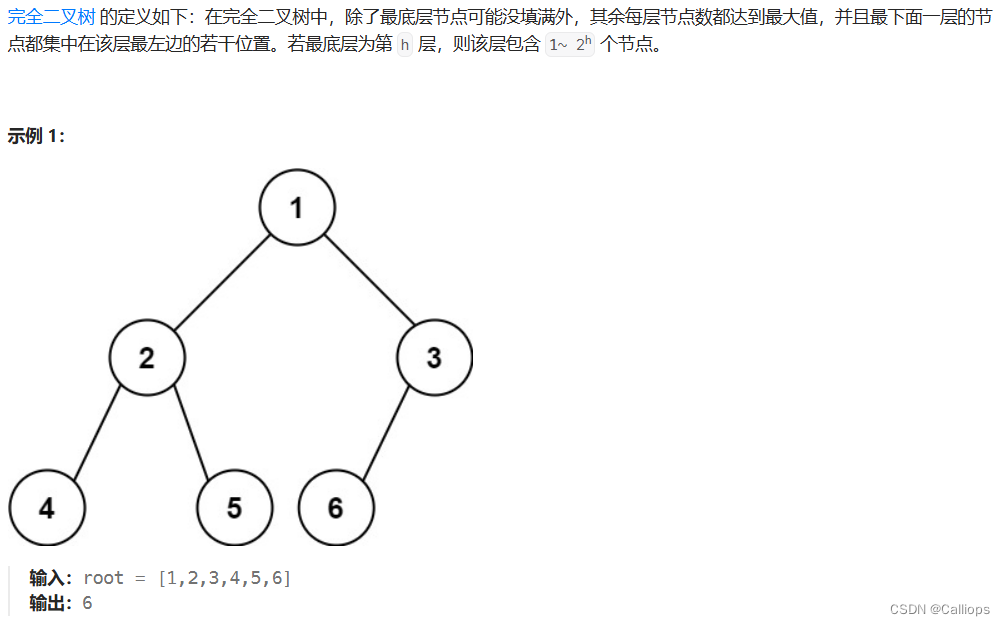
思路
方法一 当成普通二叉树来做
注意:使用后序遍历的代码是最简洁的
先序遍历:参考104题目教程里面的先序遍历写法,明显会复杂一些,为啥呢,因为需要一个全局变量来++1
单层处理逻辑:后序遍历到这个节点时,已经遍历的节点个数为它的子节点个数之和+1
递归三步走:
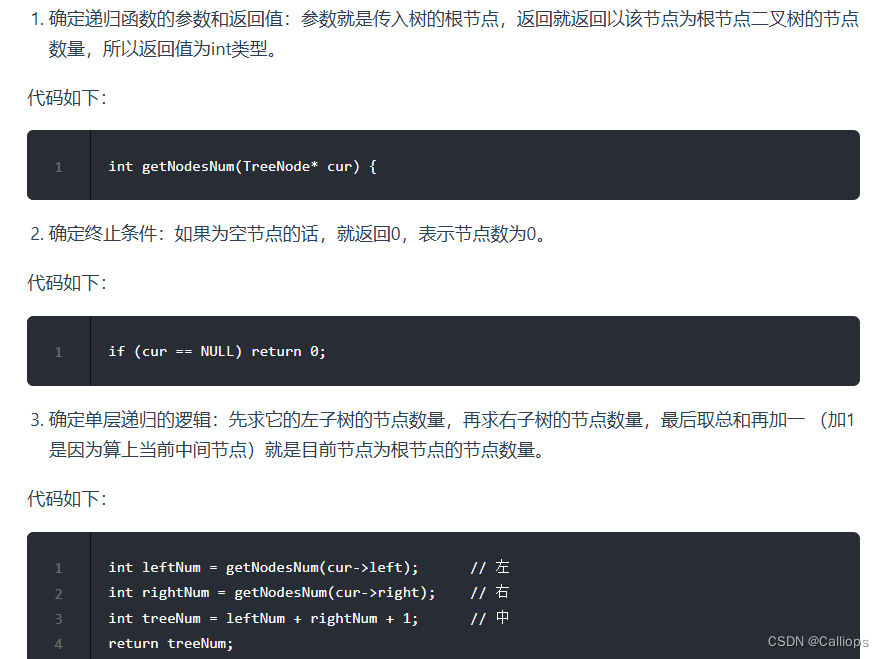
每一个都需遍历一下,时间复杂度为O(n)
class Solution(object):
def countNodes(self, root):
"""
:type root: TreeNode
:rtype: int
"""
if not root: return 0
cright = self.countNodes(root.right)
cleft = self.countNodes(root.left)
return 1+cright+cleft
方法二 利用完全二叉树的特性
首先回顾完全二叉树定义:
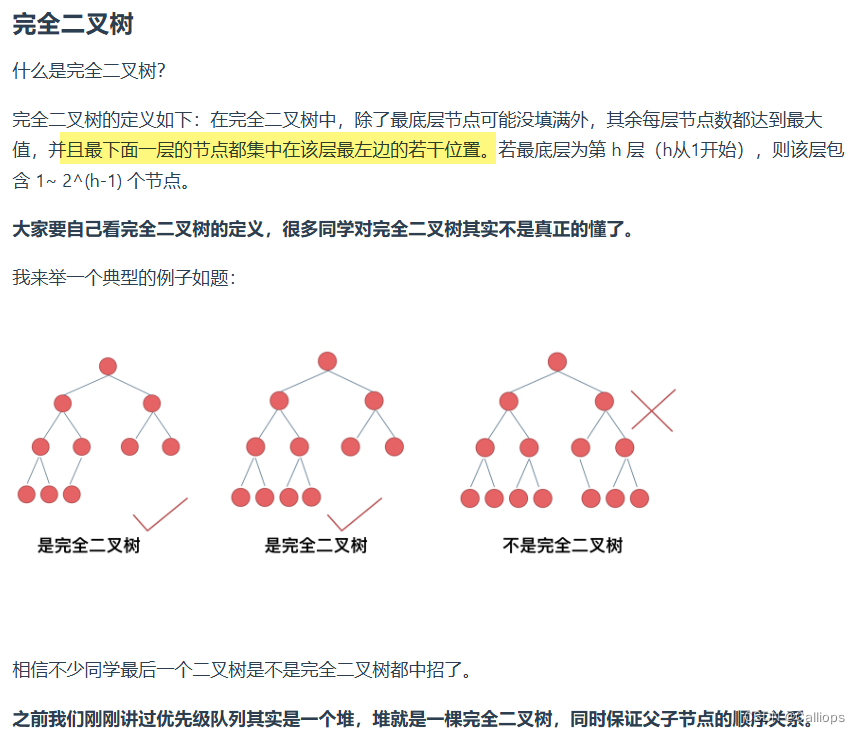
总体思路:利用满二叉树如果知道深度为n,节点个数就是2**n-1的特性,避免遍历所有的节点;
递归第三步单层处理逻辑:
- 完全二叉树只有两种情况,情况一:就是满二叉树,情况二:最后一层叶子节点没有满。
-
对于情况一,可以直接用 2^树深度 - 1 来计算,注意这里根节点深度为1。
-
对于情况二,左右孩子节点数之和加1
- 左孩子,和右孩子的计算就是递归,递归到某一深度一定会有左孩子或者右孩子为满二叉树,然后依然可以按照情况1来计算。

如何判断是否为完全二叉树:最左边一层和最右边一层节点数相同,这样就只需要遍历最外侧的就行

注意事项
-
- left和right侧边count的起始为1
- 2的阶数写为(2 << leftDepth) - 1 #注意(2<<1) 相当于2^2,所以leftDepth初始为0
class Solution(object):
def countNodes(self, root):
"""
:type root: TreeNode
:rtype: int
"""
# if not root: return 0
# cright = self.countNodes(root.right)
# cleft = self.countNodes(root.left)
# return 1+cright+cleft
if not root: return 0
#判断是否为满二叉树
left = root.left
right = root.right
left_height, right_height = 1,1# 注意这个是1
while(left):
left_height +=1
left = left.left
while(right):
right_height += 1
right = right.right
if right_height == left_height:
return 2**right_height -1
cleft = self.countNodes(root.left)
cright = self.countNodes(root.right)
return 1+cleft+cright
#还有一种技巧计算2的阶数,这时起始height要计算为0;代码如下,
class Solution:
def countNodes(self, root: TreeNode) -> int:
if not root:
return 0
left = root.left
right = root.right
leftDepth = 0 #这里初始为0是有目的的,为了下面求指数方便
rightDepth = 0
while left: #求左子树深度
left = left.left
leftDepth += 1
while right: #求右子树深度
right = right.right
rightDepth += 1
if leftDepth == rightDepth:
return (2 << leftDepth) - 1 #注意(2<<1) 相当于2^2,所以leftDepth初始为0
return self.countNodes(root.left) + self.countNodes(root.right) + 1
更加简洁的写法:完全二叉树写法2【教程里面的】
两侧同时顺着边数,直到有一条边为none,然后依据是否同时到底来判断是否为满二叉树;
class Solution: # 利用完全二叉树特性
def countNodes(self, root: TreeNode) -> int:
if not root: return 0
count = 1
left = root.left; right = root.right
while left and right:
count+=1
left = left.left; right = right.right
if not left and not right: # 如果同时到底说明是满二叉树,反之则不是
return 2**count-1
return 1+self.countNodes(root.left)+self.countNodes(root.right)
总结
比较有意思,今天都不想听申论课了。还是算法好玩。。。。可惜找不到工作