数据结构05：树与二叉树 习题01[C++]

news2024/11/24 15:33:13

   

考研笔记整理，本篇作为树与二叉树的基本概念习题，供小伙伴们参考~🥝🥝

之前的博文链接在此：数据结构05：树与二叉树[C++]-CSDN博客~🥝🥝

  • 第1版：王道书的课后习题~🧩🧩

编辑：梅头脑🌸

参考用书：王道考研《2025年 数据结构考研复习指导》

目录

🧵01 三叉树最小高度

🧵02 节点与度（一）

🧵03 节点与度（二）

🧵04 二叉树节点与度

🧵05 满二叉树节点数

🧵06 完全二叉树节点数

🧵07 满二叉树节点与编号

🧵08 顺序存储二叉树的公共祖先

🧵09 k二叉树节点

🔚结语

🧵01 三叉树最小高度

🧩题目

含有n个结点的三叉树的最小高度是多少？

📇解题思路

 高度为1的三叉树有1个结点，等比数列求和公式为：1 = 1x(3^1-1)/(3-1) = 1
 高度为2的三叉树有4个结点，等比数列求和公式为：1+3 = 1x(3^2-1)/(3-1) = 4
 高度为3的三叉树有13个结点，等比数列求和公式为：1+3+9 = 1x(3^3-1)/(3-1) = 13
 ...
 高度为n的三叉树有1+3+9+...+3^(n-1)个结点，等比数列求和公式为：n = 1+3+9+...+3^(h-1) = 1x(3^h-1)/(3-1) = (3^h-1)/2
 移项：3^h = 2*n + 1，取对数：h = log3(2*n + 1)，向上取整，即为最小高度。

🧵02 节点与度（一）

🧩题目

已知一棵度为4的树中，度为0,1,2,3 的结点数分别为14,4,3,2个，求该树的节点总数n和度为4的结点个数，并给出推导过程。

📇解题思路

 节点数 = 分支数 + 1，因为没有指向根节点的分支；
 节点数 = 14 + 4 + 3 + 2  = 23；
 度为1、2、3的分支数 = 14*0 + 4*1 + 3*2 + 2*3 = 4 + 6 + 6 = 16；
 度为4的分支数 = 总分支数 - 度为1、2、3的分支数 = 23 - 16 = 7；
 度为4的结点数 = (度为4的分支数 + 1) / 4 = (7 + 1) / 4 = 2；
 节点总数 = 度为1、2、3的结点数 + 度为4的结点数 = 14 + 4 + 3 + 2 + 2 = 25；

🧵03 节点与度（二）

🧩题目

已知一棵度为m的树中，有n1个度为1的节点，有n2个度为2的节点……有nm个度为m的节点，问该树有多少叶节点？

📇解题思路

节点数 = 分支数 + 1，因为没有指向根节点的分支；
节点数 = n0 + n1 + n2 + n3 + …… + nm；
分支数 = n0*0 + n1*1 + n2*2 + n3*3 + …… + nm*m；
代入等式，n0 + n1 + n2 + n3 + …… + nm = n0*0 + n1*1 + n2*2 + n3*3 + …… + nm*m + 1；
n0节点总数 = n1*(1-0) + n2*(2-1) + n3*(3-1) + …… + nm*(m-1) + 1 ；

🧵04 二叉树节点与度

🧩题目

 在一棵完全二叉树中, 含有n0个叶结点, 当度为1的结点数为1时, 该树的高度是多少 ? 当度为1的结点数为0时，该树的高度是多少 ?

📇解题思路

 二叉树的节点数n = n0 + n1 + n2，其中n0为叶子节点数，n1为度为1的节点数，n2为度为2的节点数；
 二叉树的节点数n = 分支数 + 1 = n1 + 2 * n2 + 1；
 联立以上两个等式，得到n0 = n2 + 1；
 又因为完全二叉树的高度h = log2(n + 1)，向上取整； 
 当度为1的结点数为1时，n = n0 + 1 +  n0 - 1 = 2 * n0，所以 h = log2[2 * n0 + 1]，向上取整；
 当度为1的结点数为0时，n = n0 + n0 - 1 = 2 * n0 - 1，所以 h = log2[2 * n0]，向上取整；

🧵05 满二叉树节点数

🧩题目

 一棵有n个结点的满二叉树有多少个分支结点和多少个叶结点?该满二叉树的高度是多少?

📇解题思路

 二叉树的节点数n = n0 + n1 + n2，其中n0为叶子节点数，n1为度为1的节点数，n2为度为2的节点数；
 满二叉树不存在n1。又因为二叉树满足 n0 = n2 + 1;
 所以，满二叉树的节点数n = 2 * n0 - 1 = 2 * n2 + 1; 节点 n0 = (n + 1) / 2， 节点 n2 = (n - 1) / 2;
 满二叉树的节点n = 2^h - 1，高度h = log2(n + 1)。

🧵06 完全二叉树节点数

🧩题目

 已知完全二叉树的第9层有 240个结点，则整个完全二叉树有多少个结点 ? 有多少个叶结点 ?

📇解题思路

 完全二叉树的第n层最多有 2^(n-1) 个结点，第9层最多有 2^8 = 256 个结点；因此，考虑到完全二叉树的性质，240个节点均为叶结点；
 但叶结点并非仅有第9层才有，第8层也有叶结点，第8层最多有 2^7 = 128 个结点，被第9层用掉 240 / 2 = 120个叶结点，还剩 8 个叶结点；整棵树共计248个叶结点，没有 n1 节点；
 完全二叉树的结点数 n = n0 + n1 + n2 = n0 + (n0 - 1) = 2 * (240 + 8) - 1 = 495 个结点；

🧵07 满二叉树节点与编号

🧩题目

一棵高度为h的满 m又树有如下性质 : 根结点所在层次为第1层，第h层上的结点都是叶结点，其余各层上每个结点都有m棵非空子树，若按层次自顶向下，同一层自左向右
顺序从1开始对全部结点进行编号，试问 :
1)各层的结点个数是多少 ?
2)编号为i的结点的双亲结点(若存在)的编号是多少 ?
3)编号为i的结点的第k个孩子结点(若存在)的编号是多少 ?
4)编号为i的结点有右兄弟的条件是什么 ? 其右兄弟结点的编号是多少 ?

📇解题思路

 1）等比数列，第i层的结点个数是 m^(i-1)；
 3）在三叉树中，节点2、3、4的双亲节点为1，节点5、6、7的双亲节点为2，即孩子节点 = (双亲节点i - 1) * 3 + 1 + k；同理，在m叉树中，第2、3、...m + 1的双亲节点为1，节点m + 2、m + 3、...2m + 1的双亲节点为2，即孩子节点 = (双亲节点i - 1) * m + 1 + k；
 2）根据3)题，第1个孩子节点 = (双亲节点i - 1) * m + 2；因此，编号为i的结点的双亲结点的编号是 ((i - 2) / m) + 1，向下取整；
 4）根据3)题，最后1个孩子节点i = (双亲节点 - 1) * m + 1；因此，编号为i的结点有右兄弟的条件是 (i - 1) % m != 0，其右兄弟结点的编号是 i + 1；

🧵08 顺序存储二叉树的公共祖先

🧩题目

已知一棵二叉树按顺序存储结构进行存储，设计一个算法，求编号分别为i和j的两个结点的最近的公共祖先结点的值。

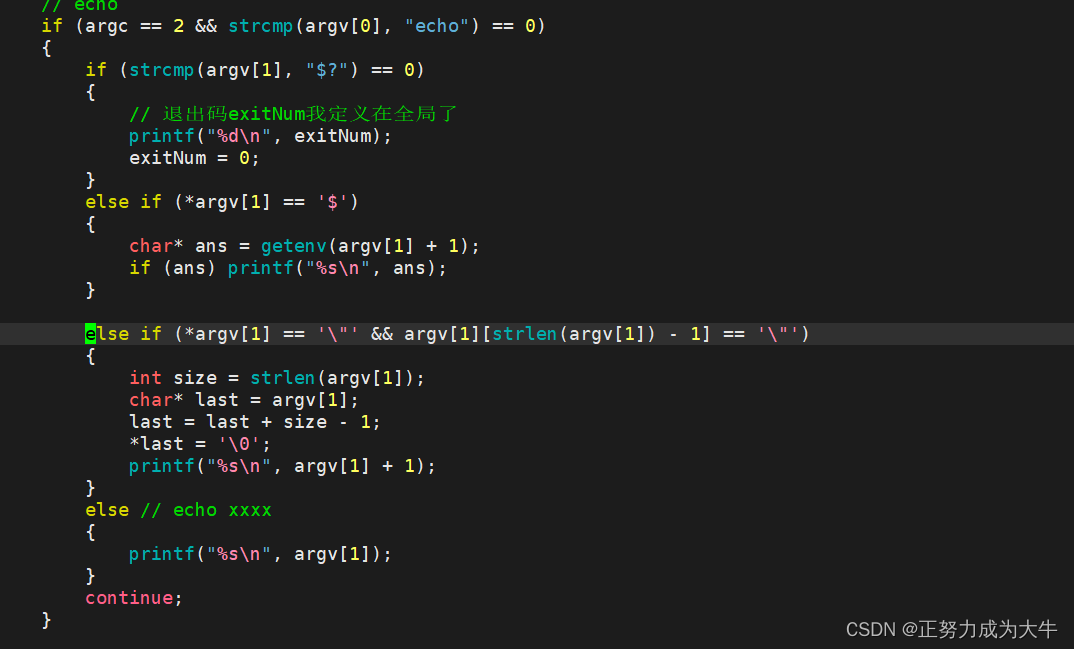
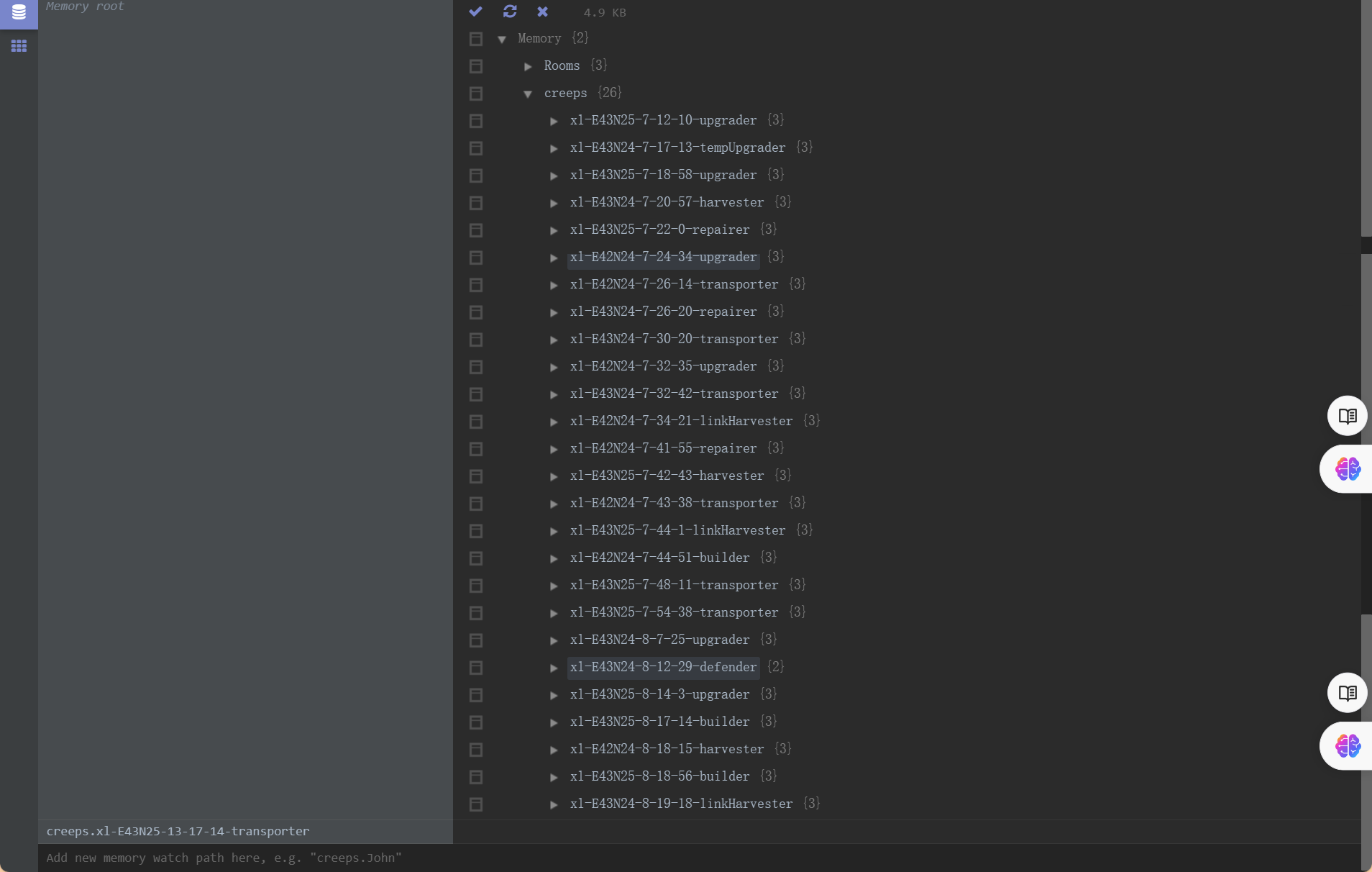
📇友情配图

我们以下的代码以这棵树为例，为了方便求祖先，这里将数组编号与内容对应一致~

以下解法的核心思路都是建立在：

  • 父节点序号 = （子节点序号 - 1）/ 2 的基础上，注意计算机运算会自动向下取整；
  • 假设树中节点没有负值，返回-1表示该节点不存在。

📇解题思路1：使用2个栈完成遍历

使用两个栈，分别存储节点 1 和 2 的祖先。

然后从两个栈的起点开始同时遍历，如果节点相同，表明该节点是二者公共祖先；否则，该节点不满足题目要求，停止循环。

这个解题代码的空间复杂度和时间复杂度都很高，完全不推荐！

⌨️解题代码

#include <iostream>
#include <vector>
#include <stack>
using namespace std;

// 二叉树的顺序存储结构
typedef struct {
	vector<int> tree;
} SqBiTree;

int find_common_ancestor(SqBiTree T, int i, int j) {
	if (i < 0 || i >= T.tree.size() || j == -1 || j >= T.tree.size()) { 
        return -1; 
    }

	// 根据节点下标，确认祖先节点的位置，并将祖先全部入栈
	int index1 = i;
	int index2 = j;
	stack<int> ancestor1;
	stack<int> ancestor2;
	do {
		ancestor1.push(T.tree[index1]);
		if (index1 > 1) { index1 = (index1 - 1) / 2; }
		else { index1 = index1 - 1; }
	} while (index1 >= 0);
	do {
		ancestor2.push(T.tree[index2]);
		if (index2 > 1) index2 = (index2 - 1) / 2;
		else { index2 = index2 - 1; }
	} while (index2 >= 0);

	// 从栈顶开始遍历，如果节点相同，继续往下遍历；如果节点不同，退出循环
	int common_ancestor = -1;
	while (!ancestor1.empty() && !ancestor2.empty()) {
		if (ancestor1.top() != ancestor2.top()) { break; }
		common_ancestor = ancestor1.top();
		ancestor1.pop();
		ancestor2.pop();
	}

	return common_ancestor;
}


int main() {
	SqBiTree T = { {0, 1, 2, 3, 4, 5, 6, 7, 8} };

	int node1 = -1, node2 = -1, result = -1;

	node1 = 7, node2 = 8;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 5, node2 = 2;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 3, node2 = 6;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 9, node2 = 3;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	return 0;
}

⌨️跑题代码

以下这个代码跑题了，传入的参数是节点值，而非节点编号，😣——

#include <iostream>
#include <vector>
#include <stack>
using namespace std;

// 二叉树的顺序存储结构
typedef struct {
	vector<int> tree;
} SqBiTree;

int find_common_ancestor(SqBiTree T, int node1, int node2) {
	// 从数组中找到 node1, node2的下标
	int locate1 = -1;
	int locate2 = -1;
	for(int i = 0; i < T.tree.size(); i++){
		if (T.tree[i] == node1) { locate1 = i; }
		if (T.tree[i] == node2) { locate2 = i; }
	}
	if (locate1 == -1 || locate2 == -1) { return -1; }

	// 根据节点下标，确认祖先节点的位置，并将祖先全部入栈
	int index1 = locate1;
	int index2 = locate2;
	stack<int> ancestor1;
	stack<int> ancestor2;
	do {
		ancestor1.push(T.tree[index1]);
		if (index1 > 1) { index1 = (index1 - 1) / 2; }
		else {index1 = index1 - 1;}
	} while (index1 >= 0);
	do {
		ancestor2.push(T.tree[index2]);
		if (index2 > 1) index2 = (index2 - 1) / 2;
		else { index2 = index2 - 1; }
	} while (index2 >= 0);

	// 从栈顶开始遍历，如果节点相同，继续往下遍历；如果节点不同，退出循环
	int common_ancestor = -1;
	while (!ancestor1.empty() && !ancestor2.empty()) {
		if (ancestor1.top() != ancestor2.top()) { break; }
		common_ancestor = ancestor1.top();
		ancestor1.pop();
		ancestor2.pop();
	}

	return common_ancestor;
}


int main() {
	SqBiTree T = { {0, 1, 2, 3, 4, 5, 6, 7, 8} };

	int node1 = -1, node2 = -1, result = -1;

	node1 = 7, node2 = 8;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 5, node2 = 2;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 3, node2 = 6;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 9, node2 = 3;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	return 0;
}

📇解题思路2：使用2个循环完成遍历

从2个节点的位置开始向祖先遍历，每次循环时，较远的节点找到其祖先，然后判断2个节点的祖先此时是否相等~

相比上一个代码，代码的数量、遍历的次数和使用的空间都减少了很多~

 ⌨️解题代码

#include <iostream>
#include <vector>
#include <stack>
using namespace std;

// 二叉树的顺序存储结构
typedef struct {
	vector<int> tree;
} SqBiTree;

int find_common_ancestor(SqBiTree T, int i, int j) {
	if (i < 0 || i >= T.tree.size() || j == -1 || j >= T.tree.size()) { 
		return -1; 
	}

	// 根据节点下标，确认祖先节点的位置
	while (i != j) {
		if (i > j)
			i = (i - 1) / 2;
		else
			j = (j - 1) / 2;
	}

	return T.tree[i];
}

int main() {
	SqBiTree T = { {0, 1, 2, 3, 4, 5, 6, 7, 8} };

	int node1 = -1, node2 = -1, result = -1;

	node1 = 7, node2 = 8;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 5, node2 = 2;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 3, node2 = 6;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 9, node2 = 3;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	return 0;
}

⌨️跑题代码

和上面的理由一样，以下这个代码跑题了，传入的参数是节点值，而非节点编号，😣——

#include <iostream>
#include <vector>
using namespace std;

// 二叉树的顺序存储结构
typedef struct {
	vector<int> tree;
} SqBiTree;

int find_common_ancestor(SqBiTree T, int node1, int node2) {
	// 从数组中找到 node1, node2的下标
	int locate1 = -1;
	int locate2 = -1;
	for(int i = 0; i < T.tree.size(); i++){
		if (T.tree[i] == node1) { locate1 = i; }
		if (T.tree[i] == node2) { locate2 = i; }
	}
	if (locate1 == -1 || locate2 == -1) { return -1; }

	// 根据节点下标，确认祖先节点的位置
	while (locate1 != locate2) {
		if (locate1 > locate2)
			locate1 = (locate1 - 1) / 2;
		else
			locate2 = (locate2 - 1) / 2;
	}

	return T.tree[locate1];
}


int main() {
	SqBiTree T = { {0, 1, 2, 3, 4, 5, 6, 7, 8} };

	int node1 = -1, node2 = -1, result = -1;

	node1 = 7, node2 = 8;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 5, node2 = 2;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 3, node2 = 6;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	node1 = 9, node2 = 3;
	result = find_common_ancestor(T, node1, node2);
	cout << "节点 " << node1 << " 与 " << node2 << " 的公共祖先是节点 " << result << endl;

	return 0;
}

🧵09 k二叉树节点

🧩题目

【2016统考真题】若一棵非空k(k >= 2)又树T中的每个非叶结点都有k个孩子，则称I为正则k叉树。请回答下列问题并给出推导过程。
1)若T有m个非叶结点，则T中的叶结点有多少个 ?
2)若T的高度为h(单结点的树h = 1)，则T的结点数最多为多少个 ? 最少为多少个 ?

📇解题思路

1) 最少是除了根节点层与底层外，每层有1个节点有k个孩子，其它 k-1 个节点是叶结点：n = m + n0 = 1 + k * m， n0 = (k - 1)*m + 1;
2) 最多是满二叉树：1 + k + k^2 + ... = (k^(h - 1) - 1)(k - 1); 最少是1 + k + k + ... = k * (h - 1) + 1;

🔚结语

博文到此结束，写得模糊或者有误之处，欢迎小伙伴留言讨论与批评，督促博主优化内容{例如有错误、难理解、不简洁、缺功能}等，博主会顶锅前来修改~😶‍🌫️

我是梅头脑，本片博文若有帮助，欢迎小伙伴动动可爱的小手默默给个赞支持一下，收到点赞的话，博主肝文的动力++~🌟🌟

同系列的博文：🌸数据结构_梅头脑_的博客-CSDN博客

同博主的博文：🌸随笔03 笔记整理-CSDN博客

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://www.coloradmin.cn/o/1660599.html

如若内容造成侵权/违法违规/事实不符，请联系多彩编程网进行投诉反馈，一经查实，立即删除！

相关文章

YOLO-World环境搭建推理测试

YOLO-World环境搭建推理测试

一、引子 CV做了这么多年&#xff0c;大多是在固定的数据集上训练&#xff0c;微调&#xff0c;测试。突然想起来一句话&#xff0c;I have a dream&#xff01;就是能不能不用再固定训练集上捣腾&#xff0c;也就是所谓的开放词汇目标检测&#xff08;OVD&#xff09;。偶尔翻…
阅读更多...
【总结】CE认证详解

【总结】CE认证详解

文章目录 CE认证 CE作用 适用范围 测试项目 一、2014/30/EU指令&#xff0c;电磁兼容&#xff08;EMC&#xff09;测试项目 二、2014/35/EU指令&#xff0c;低电压&#xff08;LVD&#xff09;测试项目 三、2011/65/EU指令&#xff0c;有害物质&#xff08;RoHS&#xff09…
阅读更多...
Linux进程间通信方式

Linux进程间通信方式

每个进程的用户空间都是独立的&#xff0c;不能相互访问。 所有进程的内核空间(32位系统3G-4G)都是共享的 应用场景 作为缓冲区&#xff0c;处理速度不同的进程之间的数据传输资源共享&#xff1a;多个进程之间共享同样的资源&#xff0c;一个进程对共享数据的修改&#xff0c…
阅读更多...
MathType2024官方版数学公式编辑器功能全面介绍

MathType2024官方版数学公式编辑器功能全面介绍

在数字化学习和科研的浪潮中&#xff0c;数学公式的编辑与展示成为了不可或缺的一部分。MathType&#xff0c;作为一款专业的数学公式编辑器&#xff0c;凭借其强大的功能和便捷的操作&#xff0c;为科研人员、教师、学生等广大用户提供了极大的便利。下面&#xff0c;我们将对…
阅读更多...
docker compose kafka集群部署

docker compose kafka集群部署

kafka集群部署 目录 部署zookeeper准备工作2、部署kafka准备工作3、编辑docker-compose.yml文件4、启动服务5、测试kafka6、web监控管理 部署zookeeper准备工作 mkdir data/zookeeper-{1,2,3}/{data,datalog,logs,conf} -p cat >data/zookeeper-1/conf/zoo.cfg<<EOF…
阅读更多...
在CentOS 7服务器及Windows 10客户端间建立并配置NFS服务

在CentOS 7服务器及Windows 10客户端间建立并配置NFS服务

在CentOS 7服务器及Windows 10客户端间建立并配置NFS服务 引言 网络文件系统(Network File System)&#xff0c;简称NFS&#xff0c;是一种分布式文件系统协议。它允许网络上的客户端机器像访问本地磁盘文件一样&#xff0c;通过网络访问服务器上的文件。在某些特定的业务场景中…
阅读更多...
【Linux】模拟实现bash（简易版）

【Linux】模拟实现bash（简易版）

&#x1f466;个人主页&#xff1a;Weraphael ✍&#x1f3fb;作者简介&#xff1a;目前正在学习c和算法 ✈️专栏&#xff1a;Linux &#x1f40b; 希望大家多多支持&#xff0c;咱一起进步&#xff01;&#x1f601; 如果文章有啥瑕疵&#xff0c;希望大佬指点一二 如果文章对…
阅读更多...
未来相遇过去：博物馆藏品管理平台的科技革新之旅

未来相遇过去：博物馆藏品管理平台的科技革新之旅

引言&#xff1a; 尊重历史&#xff0c;意味着保护其实体的载体。在博物馆这个时间的容器中&#xff0c;每一件藏品都承载着人类文明的印记&#xff0c;它们是历史的低语&#xff0c;是过去对现在的细语。在这篇文章中&#xff0c;我将带您走进博物馆的幕后&#xff0c;探究藏品…
阅读更多...
wireshark-cli工具Tshark工具使用教程

wireshark-cli工具Tshark工具使用教程

介绍 本文档基于wireshark-2.6.10/编写 tshark为wireshark工具的命令行版本呢&#xff0c; 在服务器版本服务器上&#xff0c;通过tshark工具可以实现和wireshark相同的功能。工具使用wireshark默认配置&#xff0c;对于wireshark一些常用的首选项&#xff0c;也可通过tshark…
阅读更多...
文献速递：深度学习医学影像心脏疾病检测与诊断--基于迁移学习的生成对抗网络用于静态和动态心脏PET的衰减校正

文献速递：深度学习医学影像心脏疾病检测与诊断--基于迁移学习的生成对抗网络用于静态和动态心脏PET的衰减校正

Title 题目 Transfer learning‑based attenuation correction for static and dynamic cardiac PET using a generative adversarial network 基于迁移学习的生成对抗网络用于静态和动态心脏PET的衰减校正 01 文献速递介绍 心脏正电子发射断层扫描&#xff08;PET&#xf…
阅读更多...
【ArcGIS Pro微课1000例】0059：计算地级城市之间的距离

【ArcGIS Pro微课1000例】0059：计算地级城市之间的距离

一、加载数据并符号化 1. 加载实验数据 数据加载完毕。 2. 符号化设置 点击面状数据符号，在右侧的符号系统中选择黑色轮廓。 点击点状符号，选择以个样式。 3. 标注名称 选择地级市图层，打开标注选项卡，设置标注字段为name，设置字体属性，如下所示：
阅读更多...
Screeps工程化之数量控制模块

Screeps工程化之数量控制模块

前言 将Screeps的代码进行模块化后&#xff0c;可以将各个功能进行分离&#xff0c;互相不影响&#xff0c;本文将会介绍Screeps中如何进行creep的数量控制来维持房间资源的平衡和发展。本文仅为作者本人的游戏思路&#xff0c;并不是最佳实践&#xff0c;如有更好的实现方法可…
阅读更多...
*args和**kwargs的使用

*args和**kwargs的使用

*args传入的是按照顺序的不定长度的参数列表 **kwargs传入的是不定长度的键值对
阅读更多...
effective python学习笔记_类与接口

effective python学习笔记_类与接口

用组合类实现多层结构而不用内置类型 例子&#xff1a;成绩单&#xff0c;存储学生各科成绩多个然后加权重&#xff0c;如果用字典类型会导致字典有多层嵌套结构 思想 当用内置类型如字典元组等结构出现超过二层的多层嵌套结构时&#xff0c;读起来会比较难懂&#xff0c;此时…
阅读更多...
顺序表经典算法OJ题-- 力扣27，88

顺序表经典算法OJ题-- 力扣27，88

题1&#xff1a; 移除元素 题2&#xff1a; 合并两个有序数组 一&#xff1a;题目链接&#xff1a;. - 力扣&#xff08;LetCode&#xff09; 思路&#xff1a;&#xff08;双指针法&#xff09; 创建两个变量src&#xff0c;dst 1&#xff09;若src指向的值为val&#xf…
阅读更多...
《第一行代码》第二版学习笔记（11）——最佳的UI体验

《第一行代码》第二版学习笔记（11）——最佳的UI体验

文章目录 一、Toolbar二、滑动菜单1、DrawerLayout——抽屉2、NavigationView 三、悬浮按钮和可交互提示1、FloatingActionButton——悬浮按钮2、Snackbar——提示工具3、CoordinatorLayout 四、卡片式布局1、cardView2、AppBarLayout 五、下拉刷新——SwipeRefreshLayout六、可…
阅读更多...
EDA（六）Modelsim

EDA（六）Modelsim

EDA&#xff08;六&#xff09;Modelsim ModelSim是一款由Mentor Graphics公司&#xff08;现为Siemens EDA的一部分&#xff09;开发的高性能、交互式和可扩展的HDL&#xff08;硬件描述语言&#xff09;仿真工具。它支持Verilog、SystemVerilog和VHDL等语言&#xff0c;被广泛…
阅读更多...
如何在家轻松赚钱，分享五个在家赚钱的好点子

如何在家轻松赚钱，分享五个在家赚钱的好点子

在家轻松赚钱可以考虑以下几种方式 1. 网络任务 网上可以做下百度的致米宝库&#xff0c;一个月有个一千多块钱&#xff0c;我感觉还行&#xff0c;比较适合新手小白的&#xff0c;自由操作即可。 2. 写作和翻译 如果你擅长写作或翻译&#xff0c;可以在各种写作平台或翻译平…
阅读更多...
用户体验优化uxo指的是什么？

用户体验优化uxo指的是什么？

用户体验优化(User Experience Optimization&#xff0c;简称UXO)是一种专注于改善和提升用户在使用企业产品或服务时的整体感受和体验的过程。简单来说&#xff0c;它旨在通过改进产品或服务的设计和功能&#xff0c;使用户在使用过程中感到更加愉悦、满意和高效。用户体验优化…
阅读更多...
java注解全网最细

java注解全网最细

引言 在java编程中&#xff0c;注解&#xff08;Annotation&#xff09;是一种元数据&#xff0c;它提供了关于程序代码的额外信息。注解不直接影响程序的执行&#xff0c;但可以在运行时提供有关程序的信息&#xff0c;或者让编译器执行额外的检查。 下面笔者通过循序渐进的…
阅读更多...
推荐文章
最新文章

Copyright @ 2022~2023