代码随想录-算法训练营day02【滑动窗口、螺旋矩阵】

news2024/10/5 19:11:01

专栏笔记：https://blog.csdn.net/weixin_44949135/category_10335122.html

https://docs.qq.com/doc/DUGRwWXNOVEpyaVpG?u=c71ed002e4554fee8c262b2a4a4935d8
 
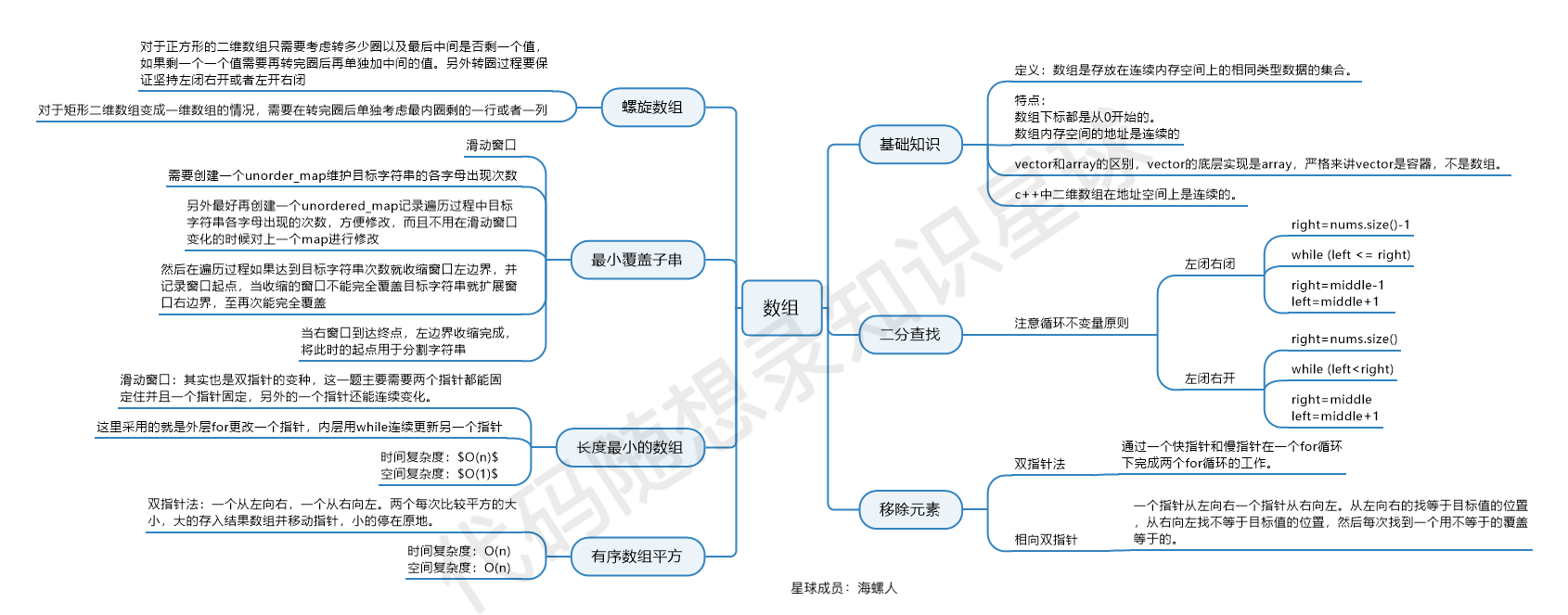
977.有序数组的平方 ，209.长度最小的子数组 ，59.螺旋矩阵II ，总结 

建议大家先独立做题，然后看视频讲解，然后看文章讲解，然后在重新做一遍题，把题目AC，最后整理成今日当天的博客

拓展题目可以先不做

 详细布置

 977.有序数组的平方 

题目建议： 本题关键在于理解双指针思想 

题目链接：https://leetcode.cn/problems/squares-of-a-sorted-array/
文章讲解：https://programmercarl.com/0977.%E6%9C%89%E5%BA%8F%E6%95%B0%E7%BB%84%E7%9A%84%E5%B9%B3%E6%96%B9.html
视频讲解： https://www.bilibili.com/video/BV1QB4y1D7ep 

 209.长度最小的子数组

题目建议： 本题关键在于理解滑动窗口，这个滑动窗口看文字讲解 还挺难理解的，建议大家先看视频讲解。  拓展题目可以先不做。 

题目链接：https://leetcode.cn/problems/minimum-size-subarray-sum/
文章讲解：https://programmercarl.com/0209.%E9%95%BF%E5%BA%A6%E6%9C%80%E5%B0%8F%E7%9A%84%E5%AD%90%E6%95%B0%E7%BB%84.html
视频讲解：https://www.bilibili.com/video/BV1tZ4y1q7XE


 59.螺旋矩阵II

题目建议：  本题关键还是在转圈的逻辑，在二分搜索中提到的区间定义，在这里又用上了。 

题目链接：https://leetcode.cn/problems/spiral-matrix-ii/
文章讲解：https://programmercarl.com/0059.%E8%9E%BA%E6%97%8B%E7%9F%A9%E9%98%B5II.html
视频讲解：https://www.bilibili.com/video/BV1SL4y1N7mV/

 总结 

题目建议：希望大家 也做一个自己 对数组专题的总结

文章链接：https://programmercarl.com/%E6%95%B0%E7%BB%84%E6%80%BB%E7%BB%93%E7%AF%87.html

0977_有序数组的平方【双指针】

0209_长度最小的子数组【滑动窗口】

0904_水果成篮

0076_最小覆盖子串

0059_螺旋矩阵2

0054_螺旋矩阵

LCR 146. 螺旋遍历二维数组

0977_有序数组的平方【双指针】

class Solution {
    public int[] sortedSquares(int[] nums) {
        for (int i = 0; i < nums.length; i++) {
            nums[i] = nums[i] * nums[i];
        }
        Arrays.sort(nums);
        return nums;
    }

    public int[] sortedSquares2(int[] nums) {//双指针法
        int left = 0, right = nums.length - 1, index = nums.length - 1;
        int res[] = new int[nums.length];
        while (left <= right) {
            if (Math.abs(nums[left]) > Math.abs(nums[right])) {
                res[index--] = nums[left] * nums[left];
                left++;
            } else {
                res[index--] = nums[right] * nums[right];
                right--;
            }
        }
        return res;
    }
}

0209_长度最小的子数组【滑动窗口】

class Solution {
    public int minSubArrayLen(int target, int[] nums) {//滑动窗口
        int sum = 0, index = 0, res = Integer.MAX_VALUE;
        for (int i = 0; i < nums.length; i++) {
            sum += nums[i];
            while (sum >= target) {
                //int len = i - index + 1;
                res = Math.min(res, i - index + 1);
                sum -= nums[index++];
            }
        }
        return res == -1 ? 0 : res;
    }

    public int minSubArrayLen2(int target, int[] nums) {//滑动窗口
        int result = Integer.MAX_VALUE;
        int sum = 0; // 滑动窗口数值之和
        int i = 0; // 滑动窗口起始位置
        int subLength = 0; // 滑动窗口的长度
        for (int j = 0; j < nums.length; j++) {
            sum += nums[j];
            //注意这里使用while，每次更新 i（起始位置），并不断比较子序列是否符合条件
            while (sum >= target) {
                subLength = (j - i + 1); // 取子序列的长度
                result = result < subLength ? result : subLength;
                sum -= nums[i++]; // 这里体现出滑动窗口的精髓之处，不断变更i（子序列的起始位置）
            }
        }
        //如果result没有被赋值的话，就返回0，说明没有符合条件的子序列
        return result == Integer.MAX_VALUE ? 0 : result;
    }

    public int minSubArrayLen3(int s, int[] nums) {//滑动窗口
        int left = 0;
        int sum = 0;
        int result = Integer.MAX_VALUE;
        for (int right = 0; right < nums.length; right++) {
            sum += nums[right];
            while (sum >= s) {
                result = Math.min(result, right - left + 1);
                sum -= nums[left++];
            }
        }
        return result == Integer.MAX_VALUE ? 0 : result;
    }
}

0904_水果成篮

class Solution0904 {
    public int totalFruit(int[] fruits) {
        int n = fruits.length;
        Map<Integer, Integer> cnt = new HashMap<Integer, Integer>();//创建一个空的哈希表

        int left = 0, ans = 0;
        for (int right = 0; right < n; ++right) {
            //把水果加入哈希表中，你可以这么看cnt.put(水果类型，水果个数)，key存储水果类型，而key对应的value存储对应的水果类型个数
            cnt.put(fruits[right], cnt.getOrDefault(fruits[right], 0) + 1);
            //当哈希表中的键值大于2时，也就是水果类型大于2时，进入while循环
            while (cnt.size() > 2) {
                //从哈希表中减去一个key==fruits[left]类型的水果，当key==fruits[left]这种类型的水果数等于0时，删除这种类型水果
                cnt.put(fruits[left], cnt.get(fruits[left]) - 1);
                if (cnt.get(fruits[left]) == 0) {
                    cnt.remove(fruits[left]);
                }
                ++left;
            }
            ans = Math.max(ans, right - left + 1);
        }
        return ans;
    }
}

0076_最小覆盖子串

class Solution {
    Map<Character, Integer> ori = new HashMap<Character, Integer>();
    Map<Character, Integer> cnt = new HashMap<Character, Integer>();

    public String minWindow(String s, String t) {
        int tLen = t.length();
        for (int i = 0; i < tLen; i++) {
            char c = t.charAt(i);
            ori.put(c, ori.getOrDefault(c, 0) + 1);
        }
        int l = 0, r = -1;
        int len = Integer.MAX_VALUE, ansL = -1, ansR = -1;
        int sLen = s.length();
        while (r < sLen) {
            ++r;
            if (r < sLen && ori.containsKey(s.charAt(r))) {
                cnt.put(s.charAt(r), cnt.getOrDefault(s.charAt(r), 0) + 1);
            }
            while (check() && l <= r) {
                if (r - l + 1 < len) {
                    len = r - l + 1;
                    ansL = l;
                    ansR = l + len;
                }
                if (ori.containsKey(s.charAt(l))) {
                    cnt.put(s.charAt(l), cnt.getOrDefault(s.charAt(l), 0) - 1);
                }
                ++l;
            }
        }
        return ansL == -1 ? "" : s.substring(ansL, ansR);
    }

    public boolean check() {
        Iterator iter = ori.entrySet().iterator();
        while (iter.hasNext()) {
            Map.Entry entry = (Map.Entry) iter.next();
            Character key = (Character) entry.getKey();
            Integer val = (Integer) entry.getValue();
            if (cnt.getOrDefault(key, 0) < val) {
                return false;
            }
        }
        return true;
    }
}

0059_螺旋矩阵2

class Solution0059 {
    public int[][] generateMatrix(int n) {
        int loop = 0;//控制循环次数
        int[][] res = new int[n][n];
        int start = 0;//每次循环的开始点(start, start)
        int count = 1;//定义填充数字
        int i, j;
        while (loop++ < n / 2) {//判断边界后，loop从1开始
            //模拟上侧从左到右
            for (j = start; j < n - loop; j++) {
                res[start][j] = count++;
            }
            //模拟右侧从上到下
            for (i = start; i < n - loop; i++) {
                res[i][j] = count++;
            }
            //模拟下侧从右到左
            for (; j >= loop; j--) {
                res[i][j] = count++;
            }
            //模拟左侧从下到上
            for (; i >= loop; i--) {
                res[i][j] = count++;
            }
            start++;
        }
        if (n % 2 == 1) {
            res[start][start] = count;
        }
        return res;
    }
}

0054_螺旋矩阵

class Solution0054 {
    public List<Integer> spiralOrder(int[][] matrix) {
        int m = matrix.length, n = matrix[0].length;
        List<Integer> res = new ArrayList<>();
        int u = 0, d = m - 1, l = 0, r = n - 1;
        while (true) {
            for (int i = l; i <= r; i++) res.add(matrix[u][i]);
            if (++u > d) break;
            for (int i = u; i <= d; i++) res.add(matrix[i][r]);
            if (--r < l) break;
            for (int i = r; i >= l; i--) res.add(matrix[d][i]);
            if (--d < u) break;
            for (int i = d; i >= u; i--) res.add(matrix[i][l]);
            if (++l > r) break;
        }
        return res;
    }
}

LCR 146. 螺旋遍历二维数组

class Solution_LCR_0146 {
    public int[] spiralArray(int[][] array) {
        if (array == null || array.length == 0 || array[0].length == 0) {
            return new int[0];
        }
        int rows = array.length, columns = array[0].length;
        boolean[][] visited = new boolean[rows][columns];
        int total = rows * columns;
        int[] order = new int[total];
        int row = 0, column = 0;
        int[][] directions = {{0, 1}, {1, 0}, {0, -1}, {-1, 0}};
        int directionIndex = 0;
        for (int i = 0; i < total; i++) {
            order[i] = array[row][column];
            visited[row][column] = true;
            int nextRow = row + directions[directionIndex][0], nextColumn = column + directions[directionIndex][1];
            if (nextRow < 0 || nextRow >= rows || nextColumn < 0 || nextColumn >= columns || visited[nextRow][nextColumn]) {
                directionIndex = (directionIndex + 1) % 4;
            }
            row += directions[directionIndex][0];
            column += directions[directionIndex][1];
        }
        return order;
    }
}