Project_Euler-44 题解

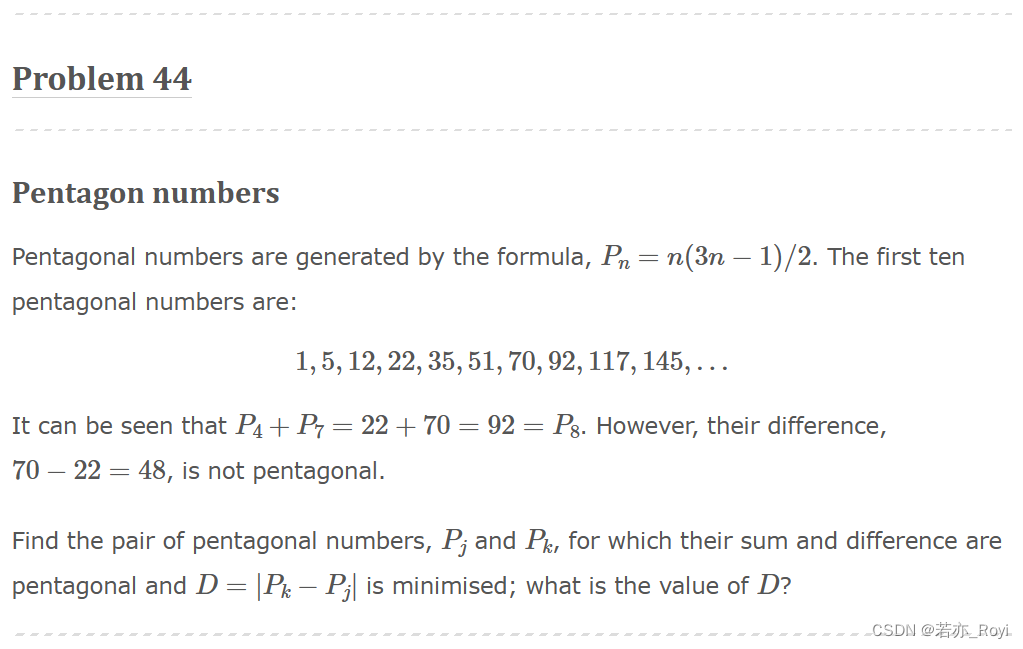
题目

思路

题目给出了一个性质，让我在对应性质的数据中找出目标值，这种问题首先想到的就是枚举。

我们可以枚举 $P_k$ ，对于每一个 $P_k$ ，我们再枚举 $P_j$，$P_j$ 从 $P_k-1$ 开始倒着往回枚举，在枚举的过程中，判断他们的和差是否均为五边形数。如果是，再与之前的已经找到的答案进行比较，如果比答案更小，说明可以取。

还有一个问题，$P_k$枚举的范围怎么确定？

假设我们枚举两个相邻的五边形数 $P_k$ 和 $P_{k-1}$ ， 会发现，他们的差值随着 $k$ 的增大而不断增大，而我们的 $P_j$ 又是从 $P_{k-1}$ 开始向前枚举的，因此，如果相邻的 $P_k$ 和 $P_{k-1}$ 已经大于目前已知的 $D$，那么我们再枚举就没有意义了，因为后面找到的答案一定大于 $D$。

对于内层循环，其实也可以使用类似的原理来做优化，如果 $P_k-P_j>D$ ，那么也不用继续枚举了，因为 $P_k-P_j$ 只会越来越大。

代码

#include <stdio.h>
#include <stdlib.h>
#include <math.h>
#include <string.h>
#include <time.h>
#include <inttypes.h>

typedef long long ll;

ll pentagonal(ll n) {
    return (n * (3 * n - 1)) >> 1;
}

ll is_pentagonal(ll x, ll n) {
    ll head = 1, tail = n, mid;
    while (head <= tail) {
        mid = (head + tail) >> 1;
        if (pentagonal(mid) == x) return 1;
        if (pentagonal(mid) < x) head = mid + 1;
        else tail = mid - 1;
    }
    return 0;
}

int main() {
    ll ans = INT32_MAX;
    ll i = 1, j = 1;
    while (pentagonal(i + 1) - pentagonal(i) < ans) {
        i += 1;
        j = i - 1;
        for (; j >= 1 && pentagonal(i) - pentagonal(j) < ans; j--) {
            if (!is_pentagonal(pentagonal(i) + pentagonal(j), 2 * i)) continue;
            if (!is_pentagonal(pentagonal(i) - pentagonal(j), 2 * j)) continue;
            printf("%lld --> %lld\n", pentagonal(j), pentagonal(i));
            ans = pentagonal(i) - pentagonal(j);
        }
    }
    printf("MIN D is %lld\n", ans);
    return 0;
}