这里写自定义目录标题
- 5.1.1 The Lagrangian
- 5.1.2 The Lagrange dual function
- 5.2 The Lagrange dual problem
- 5.2.3 Strong duality and Slater’s constraint qualification
- 5.2.3 Strong duality and Slater’s constraint qualification
- 5.5.3 KKT optimality conditions
Lagrange dual
上海交通大学 CS257 Linear and Convex Optimization
南京大学 Duality (I) - NJU
the standard form (5.1)
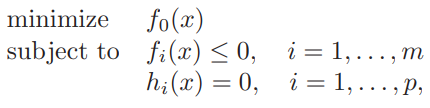
min
X
f
(
X
)
s
.
t
.
g
i
(
X
)
≤
0
,
∀
i
=
1
,
…
,
m
,
\begin{array}{l} {\mathop {\min }_{\bf{X}} \;\;f\left( {\bf{X}} \right)}\\ {{\rm{s}}.{\rm{t}}.\;{g_i}\left( {\bf{X}} \right) \le 0,\forall i = 1, \ldots ,m,} \end{array}
minXf(X)s.t.gi(X)≤0,∀i=1,…,m,
5.1.1 The Lagrangian
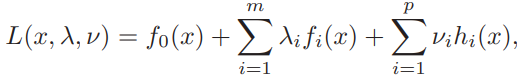
the dual variables or Lagrange multiplier vectors associated with the problem (5.1).
5.1.2 The Lagrange dual function
the minimum value of the Lagrangian
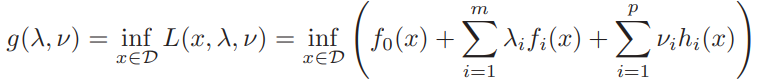
5.2 The Lagrange dual problem
the Lagrange dual problem associated with the problem (5.1).
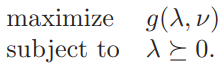
5.2.3 Strong duality and Slater’s constraint qualification

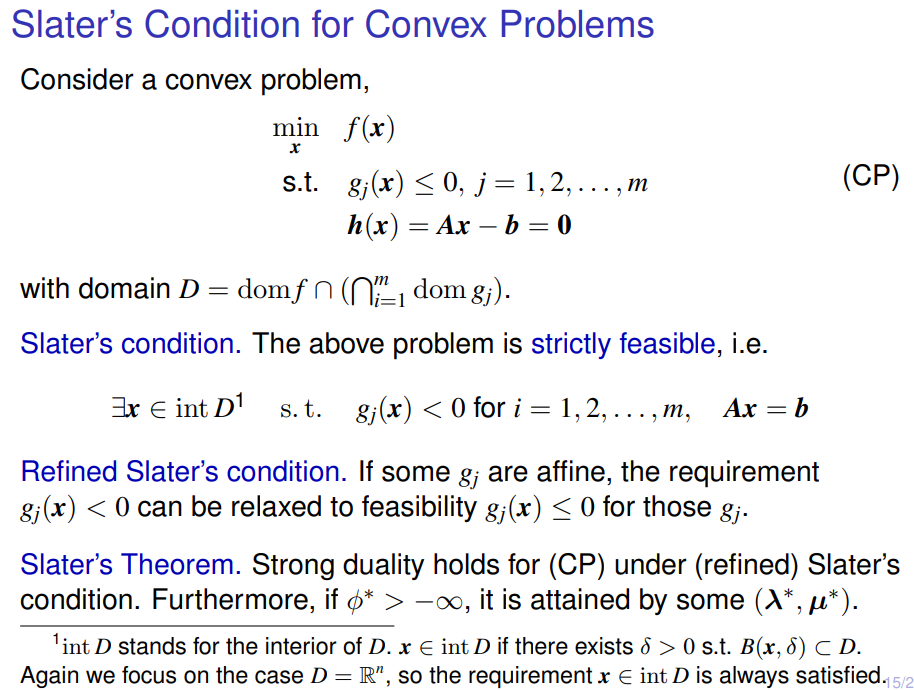
5.2.3 Strong duality and Slater’s constraint qualification
Slater’s theorem states that
strong duality holds, if Slater’s condition holds (and the problem is convex).
strong duality obtains, when the primal problem is convex and Slater’s condition holds
Slater’s Condition for Convex Problems
上海交通大学 CS257 Linear and Convex Optimization
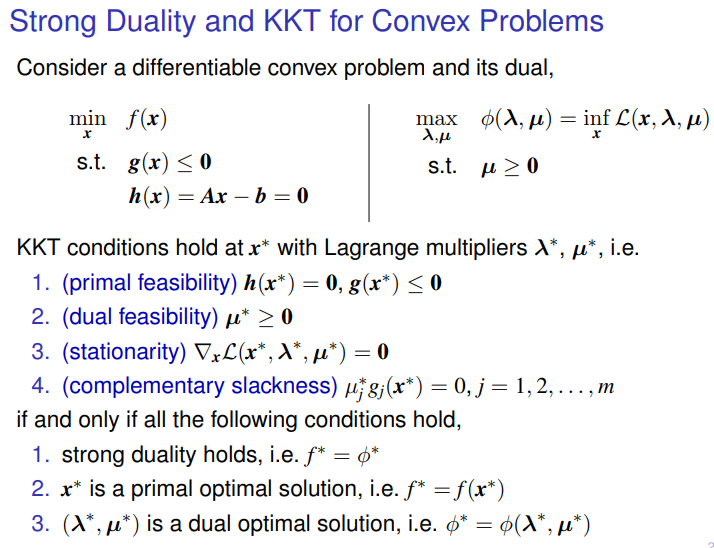
5.5.3 KKT optimality conditions
Karush-Kuhn-Tucker (KKT) conditions

for any optimization problem with differentiable objective and constraint functions for which strong duality obtains, any pair of primal and dual optimal points must satisfy the KKT conditions (5.49).