模拟

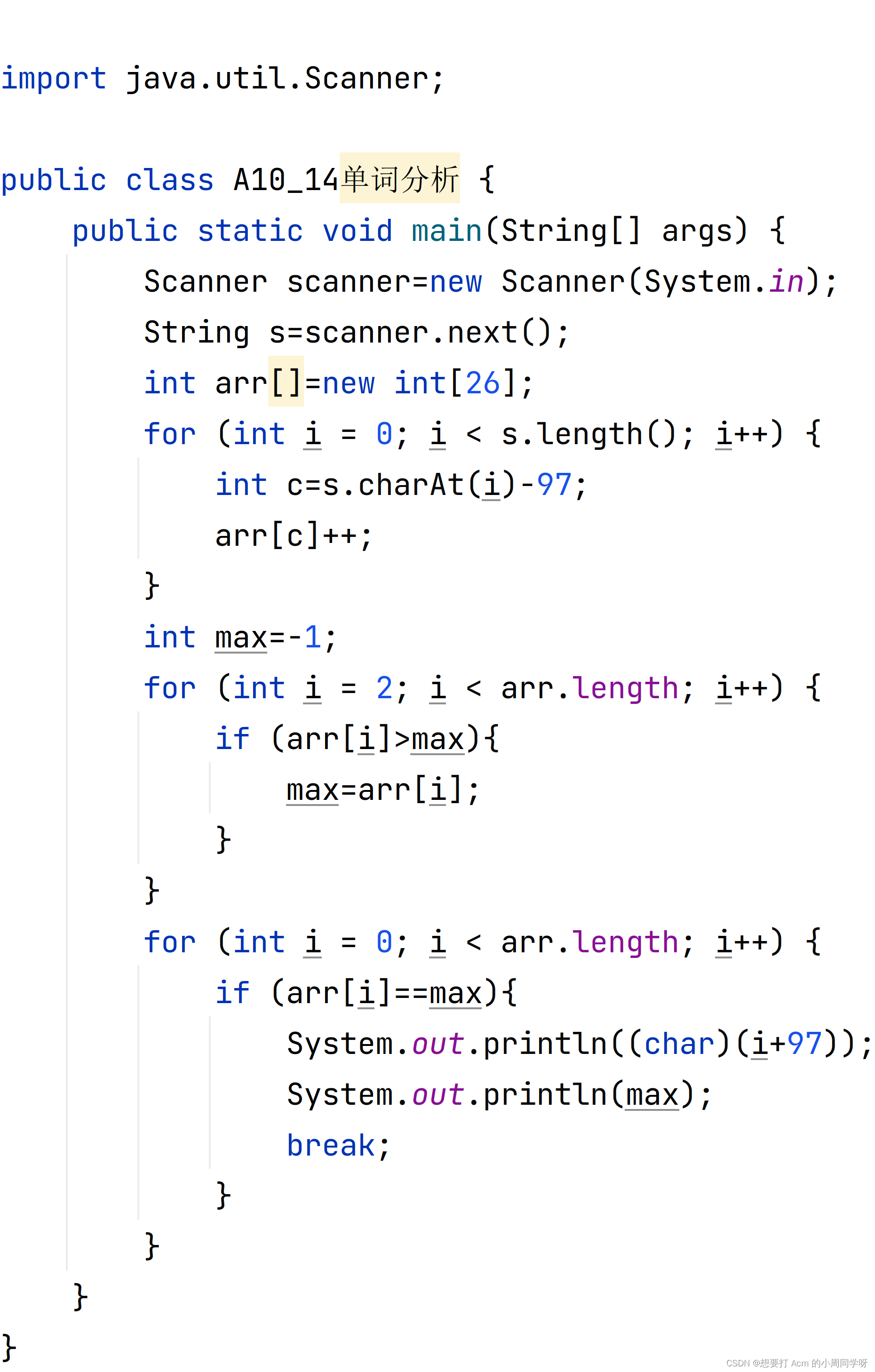
class Solution {
public:
    vector<int> lastVisitedIntegers(vector<string> &words) {
        vector<int> res;
        vector<int> li;
        for (int i = 0, n = words.size(); i < n;) {
            if (words[i] != "prev")
                li.push_back(stoi(words[i++]));
            else {
                int j = i;
                for (; j < n && words[j] == "prev"; j++) {
                    if (li.size() < j - i + 1)
                        res.push_back(-1);
                    else
                        res.push_back(li[li.size() - (j - i + 1)]);
                }
                i = j;
            }
        }
        return res;
    }
};

贪心：遍历 $groups$ ，若当前元素不等于选择的上一个位置的元素，则将当前位置加入选择的位置子序列，最终返回选择的子序列在 $words$ 对应下标的字符串序列

class Solution {
public:
    vector<string> getWordsInLongestSubsequence(int n, vector<string> &words, vector<int> &groups) {
        vector<int> ind;
        for (int i = 0; i < n; i++)
            if (ind.empty() || groups[i] != groups[ind.back()])
                ind.push_back(i);
        vector<string> res;
        for (auto x: ind)
            res.push_back(words[x]);
        return res;
    }
};

动态规划：设 $p[i]$ 为以 $i$ 结尾的满足题目所述条件的最长子序列的长度，求出 $p$ 数组后，设 $p[ind]$ 为其最大值，则从 $ind$ 开始逆序求子序列中的各个元素。

class Solution {
public:
    int comp_dis(string &a, string &b) {//计算字符串a和b的汉明距离
        if (a.size() != b.size())
            return -1;
        int res = 0;
        for (int i = 0; i < a.size(); i++)
            if (a[i] != b[i])
                res++;
        return res;
    }

    vector<string> getWordsInLongestSubsequence(int n, vector<string> &words, vector<int> &groups) {
        vector<int> p(n);
        int d[n][n];
        for (int i = 0; i < n; i++) {
            p[i] = 1;
            for (int j = 0; j < i; j++) {
                if (groups[j] == groups[i])
                    continue;
                d[i][j] = comp_dis(words[j], words[i]);
                if (d[i][j] == 1)
                    p[i] = max(p[i], p[j] + 1);//子序列中j可能是i的上一个元素
            }
        }
        int ind = 0;
        for (int i = 1; i < n; i++)
            if (p[i] > p[ind])
                ind = i;
        vector<string> res;
        while (1) {//逆序求子序列中的各个元素
            res.push_back(words[ind]);
            if (p[ind] == 1)
                break;
            for (int j = 0; j < ind; j++)
                if (groups[j] != groups[ind] && d[ind][j] == 1 && p[j] + 1 == p[ind]) {//j可以是最长子序列中ind的前一个元素
                    ind = j;
                    break;
                }
        }
        reverse(res.begin(), res.end());
        return res;
    }
};

动态规划：设 $cnt$ 表示 $nums$ 中 $vi$ 出现 $cnt[vi]$ 次，设 $p_{i,s}$ 为由 $cnt$ 中前 $i$ 个不同 $vi$ 构成的和为 $s$ 的多重集合的数目，有状态转移方程 $p_{i,s}=p_{i-1,s}+p_{i-1,s-vi}+\cdots +p_{i-1,s-vi\times cnt[vi]}$ ，类似的有 $p_{i,s-vi}=p_{i-1,s-vi}+\cdots +p_{i-1,s-vi\times (cnt[vi]+1)}$，合并一下可以得到 $p_{i,s}=p_{i,s-vi}+p_{i-1,s}-p_{i-1,s-vi\times (cnt[vi]+1)}$，另外数组中的 $0$ 需要单独处理，及答案为不考虑 $0$ 时的答案 $\times (cnt[0]+1)$。

class Solution {
public:
    using ll = long long;
    ll mod = 1e9 + 7;
    map<int, int> cnt;
    int sum_ = 0;
    int c0 = 0;

    int le(int mx) {//和不超过mx的子多重集合的数目
        int n = cnt.size();
        int p[n + 1][mx + 1];
        memset(p, 0, sizeof(p));
        p[0][0] = 1;
        auto it = cnt.begin();
        for (int i = 1; i <= n; i++) {
            int vi = it->first, ci = it->second;
            for (int s = 0; s <= mx; s++) {
                p[i][s] = p[i - 1][s];
                if (s - vi >= 0)
                    p[i][s] = (p[i][s] + p[i][s - vi]) % mod;
                if (s - vi * (ci + 1) >= 0)
                    p[i][s] = (p[i][s] - p[i - 1][s - vi * (ci + 1)]) % mod;
            }
            it++;
        }
        ll res = 0;
        for (int s = 0; s <= mx; s++)
            res = (res + p[n][s]) % mod;
        res = (res * (c0 + 1)) % mod;
        return (res + mod) % mod;
    }

    int countSubMultisets(vector<int> &nums, int l, int r) {
        for (auto x: nums) {
            if (x)
                cnt[x]++;
            else
                c0++;
            sum_ += x;
        }
        int vr = le(r);
        int vl = l != 0 ? le(l - 1) : 0;
        return ((vr - vl) % mod + mod) % mod;
    }
};